Lecture 7: P-Orbitals

Related Resources

Lecture Notes (PDF) 

• Download this transcript - PDF (English - US)

The following content is provided under a Creative Commons license. Your support will help MIT OpenCourseWare continue to offer high quality educational resources for free. To make a donation or view additional materials from hundreds of MIT courses, visit MIT OpenCourseWare at ocw.mit.edu.

PROFESSOR: All right. So, let's get started. Why doesn't everyone take 10 more seconds to answer this clicker question here.

All right. So, good. It looks like just about everyone is able to go from the name of an orbital to the state function. That's important. And we're actually going to get a little bit deeper in our clicker questions here, since when you do your problem-set it won't be quite this straight forward that you'll be answering this kind of question, but actually you'll be thinking about how many different orbitals can have certain state functions or certain orbital names.

So let's go to a second clicker question here and try one more. So why don't you tell me how many possible orbitals you can have in a single atom that have the following two quantum numbers? So let's say we have n equals 4, and n sub l equalling negative 2. How many different orbitals can you have that have those two quantum numbers in them? And this should look kind of familiar to some of the problems you may have seen on the problem-set if you started it this weekend. So this should be something you can do pretty quickly, so let's take 10 more seconds on that.

All right. OK, looks like we got the majority, which is a good start, but we having discrepancy on what people are thinking. So, let's go through this one.

So, what we're saying is that we have n equals to 4, and m sub I being equal to negative 2. If we have n equals 4, what is the highest value of l that we can have?

STUDENT: 3.

PROFESSOR: OK. We can have n 4, l 3, and then, sure, we can have m sub l equal negative 2 if l equals 3 What's the second value of l that we can have? 2. OK. So we can have this orbital here. What about l equals 1, can we have this? No, we can't. Because if l equals 1, we can not have m sub l equal negative 2, right, because the magnetic quantum number only goes from negative l to positive l here. So that means it's not possible, if we've made these stipulations in the first place, to have a case where l equals 1. So this means we can only have 2 different values of l. We already know our value of m. So now we're just counting up our orbitals, an orbital is completely described by the 3 quantum numbers. So we end up having 2 orbitals here.

All right, so hopefully if you see any other combination of quantum numbers, for example, if it doesn't quickly come to you how many orbitals you have, you can actually try to write out all the possible orbitals and that should get you started.

All right. So today we're going to finish up our discussion of the hydrogen atom. We'd started on Monday talking about radial probability distributions for the s orbitals. We'll finish that up, and then we're going to move on to talking about the p orbitals. We'll start with talking about the shape, just like we did with the s orbitals, and then move on to those radial probability distributions and compare the radial probability at different radius for p orbital versus an s orbital. And once we do that, we're actually going to move on to multi-electron atoms.

So, you might have noticed that we will have spent about 6 and 1/2 lectures just getting to the point where we have only one electron, so we're only up hydrogen so far. And you might have kind of been projecting ahead and thinking if we keep up at this pace, pretty much we would only get to carbon by the end of the semester. So I will assure you that we will not be spending 6 lectures per atom as we move forward, and in fact, what we're going to find is that by taking all the principles we've learned about the hydrogen atom and applying that to multi-electron atoms, but making a few of changes and making a few modifications to take into the fact that we're going to have electron-electron repulsions going on, we'll be able to think about any multi-electron atom using these same general ideas, and the Schrodinger equation ideas that we came up with and have looked at for the hydrogen atom.

And this is really good news because it's good to get passed carbon. I'm an organic chemist, so I love carbon, it's one of my favorite atoms to talk about, but it would be nice to get to the point of bonding and even reactions to talk about all the exciting things we can think about once we're at that point.

So, one we finish our discussion of how we think about multi-electron atoms, we can go right on and start talking about these other things.

All right. So, let's pick up where we left off, first of all we're still on the hydrogen atom from Monday. And on Monday what we were discussing was the solution to the Schrodinger equation for the wave function. And we also, when we solved or we looked at the solution to that Schrodinger equation, what we saw was that we actually needed three different quantum numbers to fully describe the wave function of a hydrogen atom or to fully describe an orbital. We didn't just need that n, not just the principle quantum number that we needed to discuss the energy, but we also need to talk about l and m, as we did in our clicker question up here.

We also talked about well, what is that when we say wave function, what does that actually mean? And first we discussed the fact that well, in terms of a classical analogy, we don't really have one for wave function, we can't really think of a way to picture wave function thinking in classical terms. But we do have an interpretation for wave function squared. And when we take the wave function and square it, that's going to be equal to the probability density of finding an electron at some point in your atom.

And that's useful in terms of seeing a general shape, but if we're actually interested in thinking about how far away that electron is from the nucleus, you can see that instead of talking about probability density, which is the probability per volume, instead it would be much more useful to talk about something called radial probability distribution, or in other words talking about the probability of finding the electron at some distance, which we define as r, from the nucleus in a spherical shell that is just infinitesimally small, infinitesimally thin at distance or at a thickness that we'll call a d r here.

So, basically what we're saying is if we take any shell that's at some distance away from the nucleus, we can think about what the probability is of finding an electron at that radius, and that's the definition we gave to the radial probability distribution. And we can look at the formula that got us here.

This is the radial probability distribution formula for an s orbital, which is, of course, dealing with something that's spherically symmetrical. It's somewhat different when we're talking about the p or the d orbitals, and we won't go into the equation there, but this will give you an idea of what we're really talking about with this radial probability distribution.

So, what we can do to actually get a probability instead of a probability density that we're talking about is to take the wave function squared, which we know is probability density, and multiply it by the volume of that very, very thin spherical shell that we're talking about at distance r. So if we want to talk about the volume of that, we just talk about the surface area, which is 4 pi r squared, and we multiply that by the thickness d r. So if we take this term, which is a volume term, and multiply it by probability over volume, what we're going to end up with is an actual probability of finding our electron at that distance, r, from the nucleus.

So, the example that we took on Monday and that we ended with when we ended class, was looking at the 1 s orbital for hydrogen atom, and what we could do is we could graph the radial probability as a function of radius here. And when we do that we can see this curve, this probability curve, where we have a maximum probability of finding the electron this far away from the nucleus. And we call that most probable radius r sub m p, or most probable radius.

And what is discussed is that for a 1 s hydrogen atom, that falls at an a nought distance away from the nucleus. And remember, a nought, that's just the Bohr radius, it's a constant -- that's all we need to worry about. We talked about the Bohr model and how that told us an exact distance. It was a classical model, right, so we could say the electron is exactly this far away from the nucleus. We can not do that with quantum mechanics, the more true picture is the best we can get to is talk about what the probability is of finding the electron at any given nucleus. And the most probable one here is that a nought.

The other thing that we looked at, which I want to stress again and I'll stress it as many times as I can fit it into lecture, because this is something that confuses students when they're trying to identify, for example, different nodes or areas of no probability. In an orbital is remember that this area right here at r equals zerio, that is not a node. We will always have r equals zero in these radial probability distribution graphs, and we can think about why that is.

At first it might be counter-intuitive because we know the probability density at the nucleus is the greatest. So the probability of having an electron at the nucleus in terms of probability per volume is very, very high. But remember that we need to multiply it by the volume here, the volume of some sphere we've defined. And when we define that as r being equal to zero, essentially we're multiplying the probability density by zero. So that's why we have this zero point here, and just to point out again and again and again, it's not a radial node, it's just a point where we're starting our graph, because we're multiplying it by r equals zero.

So, we can look at other radial probability distributions of other wave functions that we talked about. We talked about the wave function for a 2 s orbital, and also for a 3 s orbital. So, let's go ahead and think about drawing what that would look like in terms of the radial probability distribution. So what we're graphing here is the radius as a function of radial probability. And for a 2 s orbital, you get a graph that's going to look something like this.

So, again, we're starting at zero. We have one node here, and we can again define that most probable radius. And it turns out that for a 2 s orbital, that's equal to 6 times a nought.

So when we think about what it is that this radial probability distribution is telling us, it's telling us that it is most likely that an electron in a 2 s orbital of hydrogen is six times further away from the nucleus than it is in a 1 s orbital. So another way to say that is, in a sense, if we're thinking about the excited state of a hydrogen atom, the first excited state, or the n equals 2 state, what we're saying is that it's actually bigger than the ground state, or the 1 s state of a hydrogen atom. And when we say bigger, remember this is not a classical description we're talking about. We are talking about probability, but what we're saying is that most probable radius is further away from the nucleus.

So we can also look at this in terms of the 3 s orbital. And in this case, we have a graph that looks something like this. So you can draw that into your notes. And again, we can define what that most probable radius is, that distance at which we're most likely to find an electron. And in the case of the 3 s orbital, that's going to be equal to 11 . 5 times a nought.

So again, what we're saying here is that it is most likely in the 3 s orbital that we would find the electron 11 and 1/2 times further away from the nucleus than we would in a around state hydrogen atom.

And I just want to point out here in terms of things that you're responsible for, you should know that the most probable radius for a 1 s hydrogen atom is equal a nought. And you should know that a 2 s is larger than that, and a 3 s is even larger, and of course, hopefully as we go to 4 and 5, you would be able to guess that those are going to get even larger. But you're not responsible for knowing specifically that it's 11 . 5 times greater. You just need to know the trend there.

Another thing to point out in these two graphs is that we do have nodes, and we figured out last time, we calculated how many nodes we should have in a 2 s orbital. And in terms of radial nodes, we expect to see one node. And how many nodes do you see in the 3 s orbital? two, good. I'm glad to hear that no one counted this r equal zero as a node. So we expect to see two nodes right here in the 3 s orbital. And we can calculate that with the formula that we used, which was just n minus l minus 1 equals the number of nodes. Or we could just look at the radial probability distribution itself and see how many nodes there are.

So if we're looking at these two situations here and we're actually thinking of them from a more classical standpoint, which is natural for us to do because we live our lives in the every day world, not thinking about things on the atomic size scale all the time, most of us. So, for example, if we were to look at this 3 s orbital here, you might have the question of how this can be, because we're saying that, for example, we have probability of having an electron here, an electron can also be way out at this radius here. But what we're saying is there's a node here, so that there's no probability of finding an electron between those two points.

So you can think of it, if we were to just think of it as a straight line that we were going across, essentially what we're saying is that we're getting from point a to point c without ever getting through point b. So, that can be a little bit confusing for us to think about, and when it's a very good question you might, in fact, say well, maybe there's not zero probability here, maybe it's just this teeny, teeny, tiny number, and in fact, sometimes an electron can get through, it's just very low probability so that's why we never really see it. And in fact, that's not the answer. The answer is, in fact, there is zero, absolutely zero probability of finding a electron here. So basically we're saying yes, we can go from point a to point c without ever going through point b. That might seem confusing if you're thinking about particles, but remember we're talking about the wave-like nature of electrons.

So, the quantum mechanical interpretation is that we can, in fact, have probability density here and probability density there, without having any probability of having the electron in the space between. And you can think about that if you think about a standing wave, for example, where you can have amplitude at many different values of x, so an amplitude at many different distances, but you also have areas where there is a 0 amplitude. So, remember this makes sense if you just think of it as a wave and forget the particle part of it for right now, because that would be very upsetting to think about and that's not, in fact, what's going on, we're talking about quantum mechanics here.

Yes.

STUDENT: [INAUDIBLE]

PROFESSOR: Oh, I'm sorry. So it's n minus l minus 1. So here we have 3 minus l equals 0, because it's an s orbital, minus 1, so we have two radial nodes here.

OK. So let's actually go to a clicker question now on radial probability distributions. So I mentioned you should be able to identify both how many nodes you have and what a graph might look like of different radial probability distributions. So here, what I'd like you to do is identify the correct radial probability distribution plot for a 5 s orbital, and also make sure that it matches up with the right number of radial nodes that you would expect.

All right. Let's take 10 more seconds on that, this should be a quick identification for us to do.

All right. So it looks like 82% got the correct answer here. So, you should know that there's four radial nodes, right, we have 5 minus 1 minus l -- is there a question?

STUDENT: [INAUDIBLE]

PROFESSOR: It is very difficult for me to draw graphs on the computer. That's a good point, I'm sorry. This was my best attempt at hitting zero and not having the graph go down there. I'm not the most gifted at drawing on the computer. So yes, it should be zero at zero, but I made the line too thick. So, assuming -- if anyone got it wrong because of that, that's my apologies, that's my fault. But you should see that there are four radial nodes here since we have a 5 s orbital. And also that we know that the zero does not count as a node, if per se I actually had managed to hit zero in drawing that, so the correct answer would be the bottom one there.

So, you should be able to generally identify and draw the general form of these radial probability distributions. Obviously we don't expect you to know exactly what the distances are, but you should be able to compare them relatively.

Yes?

STUDENT: [INAUDIBLE]

PROFESSOR: No, they actually don't, and when you graph it all out. You can see this if you look at some examples in your book, actually. So this doesn't fall, for example, at 6 a nought, but that's a really good question. And the trend always is that the probability gets smaller with each of the peaks as you're drawing them.

All right. So we can switch back to our notes. So we got our clicker question set there.

And so now we can move on to thinking about p orbitals, we now have two ways to talk about p orbitals. We can talk about the wave function squared, the probability density, or we can talk about the radial probability distribution. So when we talk about p orbitals, it's similar to talking about s orbitals, and the difference lies, and now we have a different value for l, so l equals 1 for a p orbital, and we know if we have l equal 1, we can have three different total orbitals that have sub-shell of l equalling 1. So we can have, if we have the final quantum number m equal plus 1 or minus 1, we're dealing with a p x or a p y orbital. Remember, we don't do a one-to-one correlation, because p x and p y are some linear combination of the m plus 1 and m minus 1 orbital. And if we talk about m equals 0, we're looking at the p z orbital.

And the significant difference between s orbitals and p orbitals that comes from the fact that we do have angular momentum here in these p orbitals, is that p orbital wave functions do, in fact, have theta and phi dependence. So they do have an angular dependence that we're talking about.

And what I'm showing here is not on your notes, if you're interested you can look it up in your book. This is a table that's directly from your book, and what it's just showing is the wave function for a bunch of different orbitals. I mentioned last time that there was this list in your book. And what I want to point out here is this angular dependence for the p orbitals for the l equals 1 orbital. So, first, if I point out when l equals 0, when we have an s orbital, what you see is that angular part of the wave function is equal to a constant.

So, remember we can break up the total wave function into the radial part and the angular part. When we look at this angular part, we see that it's always the square root of 1 over 4 pi, it doesn't matter what the angle is, it's not dependent on the angle.

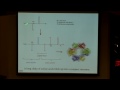
In contrast when we're looking at a p orbital, so any time l is equal to 1, and you look at angular part of the wave function here, what you see is the wave function either depends on theta or is dependent on both theta and phi. So we do, in fact, have a dependence on what the angle is of the electron as we define it in the orbital. So what this means is that unlike s orbitals, p orbitals are not spherically symmetrical -- they don't have the exact same shape at any radius from the nucleus. And these shapes of p orbitals probably do look familiar to you, most of you, I'm sure, have seen some sort of picture of p orbitals before.

So what I want to point out about them is that they're made up of two nodes, and what you can see is that nodes are shown in different colors here and those are different phases. Sometimes you see this written when you see p orbitals, one is written as plus, one is written in minus. That's not a positive and negative charge, that's actually a phase, and that arises from the wave equation. Remember when we have waves we can have positive or a negative amplitude.

When we talk about p orbitals the phase of the orbital becomes important once we talk about bonding, which hopefully you were happy to hear at the beginning of class we will get to soon. And it turns out that when you constructively have two p orbitals interfere, and when I say constructively, I mean they're both either positive or they're both the negative lobes, that's when you got bonding. Whereas if the phases where mismatched, you would not get bonding. So, that's going to be important later when we get to bonding, but just take note of it now, we have two nodes, each with a separate phase -- or we have two lobes, excuse me, each with a separate phase.

And when we look at this, it's actually split by what's called a nodal plane, which is pointed out in light orange here on this picture, but what we just mean is that there is this whole plane that separates the two lobes where there is absolutely no electron density. So, the wave function at all of these points in this plane is equal to zero, so therefore, also the wave function squared is going to be equal to zero.

So, if we say that in this entire plane we have zero probability of finding a p electron anywhere in the plane, the plane goes directly through the nucleus in every case but a p orbital, so what we can also say is that there is zero probability of finding a p electron at the nucleus.

So, again we can use these probability density plots, which are just a plot of psi squared, where the density of the dots is proportional to the density, the probability density, at that point. So what we can say is look at each of these separately, so if we start with looking at the 2 p z orbital, the highest probability of finding an electron in the 2 p z orbital, is going to be along this z-axis. We can see that right here.

And in terms of thinking about the phase of this p orbital, the phase is going to be positive anywhere where z is positive. So we would say we have a positive phase here and a negative phase there. Remember, that's going to become important when we talk about bonding, we don't need to worry about it too much right now.

We can also think about where the nodal plane is in this p z orbital, so how would we define the nodal plane here? What would the nodal plane be? So, it's the x-y plane, you can see there's no electron density anywhere there. And similarly, actually, if we're looking at our polar coordinates here, what we see is it's any place where theta is equal to 0 is what's going to put up on the x-y plane. So another way to define the nodal plane is where theta is equal to 90 degrees.

So let's look now at the 2 p x orbital. This is the probability density map, so we're talking about the square here. The highest probability now is going to be along the x-axis, so that means we're going to have a positive wave function every place where x is positive. What is the nodal plane in this case? Um-hmm. So, it's going to be the y z nodal plane, or in other words, we can say it's any place where phi is equal to 90 degrees. So you can see if you take phi, and you move it over 90 degrees, we're right here in the y z plane. Anywhere where that's the case we're going to have no probability density of finding an electron.

And finally, we can look at the 2 p y, so the highest probability is going to be along the y-axis. It's going to be positive in terms of its wave function or in terms of its phase anywhere where y is positive. And the nodal plane's going to be in the x z plane, or again, anywhere where phi is going to be equal to 0, that takes us to the x z plane.

So, let me get a little bit more specific about what we mean by nodal plane and where the idea of nodal plane comes from, and nodal planes arise from any place you have angular nodes. So we talked about radial nodes when we're doing these radial probability density diagrams here. You can also have angular notes, and when we talk about an anglar node, what we're talking about is values of theta or values of phi at which the wave function, and therefore, the wave function squared, or the probability density are going to be equal to zero.

So, you remember from last time radial nodes are values of r at which the wave function and wave function squared are zero, so the difference is now we're just talking about the angular part of the wave function. And, in fact, these are the only two types of nodes that we're going to be describing, so we can actually calculate both the total number of notes and the number of each type of node we should expect to see in any type of orbital. And our equation for total nodes is just the principle quantum number minus 1. And when we talk about angular nodes, the number of angular nodes we have in an orbital is going to be equal to l. So that's why we saw, for example, in the p orbitals we had one angular node in each p orbital, because l is equal to 1 there.

And we talked about the equation you can use for radial nodes last time, and that's just n minus 1 minus l. You can go ahead and use that equation, or you could figure it out every time, because if you know the total number of nodes, and you know the angular node number, then you know how many nodes you're going to have left. So you don't really have to memorize that.

So, let's go ahead and just do a few of these. They're pretty straight forward to do and it gives us an idea what kind of nodal structure we can expect it an orbital. So for a 2 s orbital, how many total nodes will we have? Yup, I heard one, so 2 minus 1, one total node. Angular nodes, we're not going to have any of those, we'll have zero, l equals 0, so we have zero angular nodes. And in terms of radial nodes, we have 2 minus 1 minus 0, so what we have is one radial node.

So, what you find with the s orbital, and this is general for all s orbitals is that all of your nodes end up being radial nodes. That has to be the case because l equals 0 for s orbitals.

Let's look now at a p orbital, so how many total nodes do we have here? Yup, so one total node, 2 minus 1 is 1, and that means since l is equal to 1, we have one angular nodes, and that leaves us with how many radial nodes? Yup, zero radial nodes. So, for a 2 p orbital, all the nodes actually turn out to be angular nodes.

So, let's have you try one more, if we can switch over and talk about a 3 d orbital. So, I'm asking very specifically about radial nodes here, how many radial nodes does a hydrogen atom 3 d orbital have? So, you can go ahead and take 10 seconds on that.

All right. So most of you got that, though there is this little sub-set we have thinking that we have one, so let's actually write this out here. So if we have a 3 d orbital, we're talking about n minus l minus 1, what is n equal to? What is l equal to? OK. and 1 is equal to 1. So, it turns out that we have zero nodes that we're dealing with when we're talking about a 3 d orbital.

OK. So we should be able to figure this out for any orbital that we're discussing, and when we can figure out especially radial nodes, we have a good head start on going ahead and thinking about drawing radial probability distributions. We did it for the s orbitals, we can also do it for the p, we can do it for the d. All we have to figure out is how many nodes we're dealing with and then we can get the general shape of the graph here.

So, let's actually compare the radial probability distribution of p orbitals to what we've already looked at, which are s orbitals, and we'll find that we can get some information out of comparing these graphs. So if we draw the 2 p orbital, what we just figured out was there should be zero radial nodes, so that's what we see here. The other thing that I want you to notice, is if you look at the most probable radius, for the 2 s orbital it's actually out further away from the nucleus than it is for the 2 p orbital. So what we can say here is that the 2 p is less than or smaller than the 2 s orbital.

So think about what that means, we're, of course, not talking about this in classical terms, so what it means if we have an electron in the 2 p orbital, it's more likely, the probability is that will be closer to the nucleus than it would be if it were in the 2 s orbital.

We can also take a look the 3 s, which we have looked at before, and we figured out that that should have two radial nodes. We can look at the 2 p, which should have one radial node, and we just figured it out for the, excuse me, for the 3 p has one radial node, and for the 3 d here, we should have zero radial nodes, we just calculated that. So again, what we see is the same pattern where the most probable radius, if we talk about it in terms of the d, that's going to be smaller then for the p, and the 3 p most probable radius is going to be closer to the nucleus than it is for the 3 s most probable radius that we're looking at.

So, there are 2 different things that we can compare when we're comparing graphs of radial probability distribution, and the first thing we can do is think about well, how does the radius change, or the most probable radius change when we're increasing n, when we're increasing the principle quantum number here? So, from going from the shell of n equals 2, let's say, to the shell of n equals 3. And what we find is we're going from about or exactly a 6 a nought here, to almost three times that when we're going from 2 s to 3 s. So we say if n increases, the orbital size is also going to increase. And when we talk about size, I'm again just going to say the stipulation that we're talking about, probability -- we're not talking about an absolute classical concept here, but in general we're going to picture it being much further away from the nucleus as we move up in terms of n.

The other thing that we took note as is what happens as l increases, and specifically as l increases for any given the principle quantum number. So if we're keeping n the same, we look and what we saw was that size actually decreases as we increase the value of l.

So, I want to contrast that with another concept that seemed to be opposing ideas, and that is thinking about not how far away the most probable radius is, but thinking about how close an electron can get to the nucleus if it's actually in that orbital. And what we'll find is that we actually see the opposite. So if we compare l increasing here, so a 3 s to a 3 p to a 3 d, what we find is that it's only in the s orbital that we have a significant probability of actually getting very close to the nucleus. So, if I kind of circle where the probability gets somewhat substantial here, you can see we're much closer to the nucleus at the s orbital than we are for the p, then when we are for the d.

So, the size still for an s orbital is larger than for a d orbital, but what we say is that an s electron can actually penetrate closer to the nucleus. There's some probability that it can get very, very close the nucleus, and that probability is actually substantial.

A kind of consequence of this is if we're thinking about a multi-electron atom, which we'll get to in a minute where electrons can shield each other from the pull of the nucleus, we're going to say that the electrons in the s orbitals are actually the least shielded. And the reason that they're the least sheilded is because they can get closest to the nucleus, so we can think of them as not getting blocked by a bunch of other electron, because there's some probability that they can actually work their way all the way in to the nucleus. So, this is a concept that's going to become really important. Soon when we're talking about multi-electron atoms, and I just want to introduce it here, that it is sort of opposing ideas that even though the s is the biggest and it's most likely that the electron's going to be furthest away from the nucleus, that's also the orbital in which the electron can, in fact, penetrate closest.

All right. So I think we are, in fact, ready to move on to multi-electron atoms, and what happens is when we solved the relativistic version of the Schrodinger equation and we're discussing more than one electron, we actually have a fourth quantum number that falls out and that we need to deal with and this is called the electron spin quantum number. And I promise, this is the last quantum number that we'll be introducing.

And this spin magnetic quantum number we abbreviate as m sub s, so that's to differentiate from m sub l. And when you solved the relativistic form of the Schrodinger equation, what you end up with is that you can have two possible values for the magnetic spin quantum number. You can have it equal to plus 1/2, and that's what we call spin up, or you can have it equal to minus 1/2, which is what we call spin down.

So, there's two kind of cartoons shown here that give you a little bit of an idea of what this quantum number tells us. And this spin is an intrinsic quality of the electron, it's a property that is intrinsic in all particles, just like we would say mass is intrinsic or charge is intrinsic. Spin is also an intrinsic property. One way to think about it, if we want to use a classical analogy, which often helps to give us an idea of what's going on, is the spin of an electron, we can picture it rotating on its own axis. So that's kind of what's shown in these pictures here. So you can see, if it's spinning on its own axis in this direction we'd call it spin up, where as this way it would be what we call spin down.

So, it turns out there's not actually a good classical analogy for spin, we can't really think of it like that, but if that helps give you an idea of what's going on here then it's valuable maybe to consider it spinning on its own axis, even though that's not technically what's exactly happening here. But the reason that I like that analogy is that it points out a very important part of spin, and that's the idea that it's a description of the electron. It is not dependent on the actual orbital. So we can completely describe an orbital with just using three quantum numbers, but we have this fourth quantum number that describes something about the electron that's required for now a complete description of the electron, and that's the idea of spin. So, we need to actually add on this fourth quantum number, and it's either going to be plus 1/2 or negative 1/2.

So, we can talk a little bit actually, because it's an interesting story about where the idea of spin came from, and it was actually first proposed by two very young scientists at the time, George Uhlenbeck shown here, and Samuel Goudsmit who's here, and they're with a friend, and I can't remember who that is, but he did not have anything to do with discovering spin. And what you can see in this picture is that these are actually, they're pretty young guys in this picture. I think this is taken about two years after they discovered the fourth quantum number. So hopefully, you can picture yourself at this age in a similar situation with an anonymous friend and think this is something, kind of observations maybe you can make as well.

And what they were doing when they discovered that there must be this fourth quantum number is they were looking at the emission spectrum of sodium, and, so specifically, they were looking at the frequencies, and if we think about the frequencies of sodium, it was already known at this time that you could calculate what those would be based on the difference in the energy levels -- this happened in about 1925. So they actually knew exactly what they were expecting. So, let's say they were expecting to see one certain frequency or one line in the spectrum at this point here. It turns out that what they actually observed, so this is the actual of what they saw, is if they were expecting their line at some given frequency, which I'll show by this dotted line here, what they actually saw was two lines, and one was just the teeniest tiniest bit of a higher frequency than what was expected, and one was just, just below what they expected. And if we're talking about things in spectroscopy terms here, this is what we call a doublet. So it's centered at this frequency that was expected, but it's actually split into two different frequencies.

And this was an amazing observation that they made. They were totally surprised and excited, and they were thinking how could this happen, where did we got this split doublet from. And what they could come up with, what they reasoned, is that there must be some intrinsic property within the electron, because we know that this describes the complete energy of the orbital should give us one single frequency. And that the fact that it split into two was telling them that there must be some new property to the electron, and what we call that now is either being spin up or spin down. But at the time, they didn't have a well-formed name for it, they were just saying OK, there's this fourth quantum number, there's this intrinsic property in the electron.

So, where the story gets kind of unfortunate, but also a little bit more interesting is the fact that well, they did publish what they observed, and they did write that up. They put it in a pretty low impact paper. I think it was in French, so it wasn't really hitting all the scientific community of the time in any major way. And they didn't put their explanation of what they thought was going on, it just sort of was observing what they saw. These were very young scientists, of course, so what you would expect that they would do, which makes sense, is go to someone more established in their field, because they have the completely radical revolutionary idea, let's just run it by someone before we go ahead and publish this paper that makes this huge statement about this fourth quantum number. So the person they chose to talk to, and I think it was just Goudsmit that went to him and discussed it is Wolfgang Pauli, which is shown here. So how many before five minutes ago had heard the name Goudsmit? All right, a couple. OK, cool. How about Pauli, like the Pauli exclusion principle? Hmm, OK. So, Pauli seems to be getting a little bit of fame that you'll see in a second here.

So, it turns out they go and they discuss their idea with Pauli, and what Pauli tells them is that this idea is ridiculous, that it's rubbish, if they go ahead and try to publish this their scientific careers are ruined. They can pretty much pack it up and go home, because everyone's going to think they're ridiculous, no one will believe what they say, and it's a stupid idea anyway, is basically the gist of this conversation. And in chemistry, just like in any discipline, you have all types of scientists, but also all types of personalities, and unfortunately Pauli had a personality that was known for, first of all, being very arrogant, but also the very unfortunate trait of taking other people's scientific ideas as his own.

And as the story goes, as Goudsmit was leaving and the door with slamming, Wolfgang Pauli was already writing down this idea into a scientific paper of the idea of a fourth quantum number. And, in fact, he did make some more strides, he was a brilliant thinker, maybe he put it more articulately than those two younger scientists could have. But now, it has come to light that they are the ones that do get credit for first really coming up with this idea of a spin quantum number, and it's interesting to think about how the politics work in different discoveries, as well as the discoveries themselves. You see that a lot with Nobel prizes, there's usually a nice little scandal, a nice little interesting story behind who else was responsible for the Nobel Prize-worthy discovery.

So, here, Pauli came out on top, we say, and he's known for the Pauli exclusion principle, which tells us that no two electrons in the same atom can have the same four quantum numbers.

So let's just think exactly what this means, and that means that if we take away function and we define it in terms of n, l and m sub l, what we're defining here is the complete description of an orbital. In contrast, if we're taking the wave function and describing it in terms of n, l, m sub l, and now also, the spin, what are we describing here? An electron. So now we have the complete description of an electron within an orbital.

So, that's an important distinction to make -- what three quantum numbers tell us, versus what the fourth quantum number can fill in for us in terms of information.

So what that means is that we're limited in any atom to having two electrons per orbital, right, because for any orbital we can either have a spin up electron, a spin down electron, or both.

So, if we look at neon just as an example, neon has ten electron in it, and once we look at all the orbitals written out here, this is probably a familiar thing for you to look at, but it's important to think about why, in fact, we don't just put all ten electrons, why wouldn't they just want to go in that ground state, that lowest state, right? That should be the most stable, the lowest energy orbital for it to be in, and the reason they can't do that is because of the Pauli exclusion principle -- the idea that all of the electrons have to have a different set of four quantum numbers, so only two of them can have the same set of three quantum numbers here, because for m sub s, we're only left with two options.

So let's try a clicker question and thinking about the Pauli exclusion principle. It might look a little bit similar to a question we just saw, but hopefully you'll find that it is, in fact, not the same question. So you can go ahead and take 10 seconds on that.

OK, great. So, most of you recognize that there are four different possibilities of there's four different electrons that can have those two quantum numbers. Actually the easiest way is probably to bring this down here. And the next highest percentage of you thought that we still only had two. So, remember we solved this problem earlier in the class, but we were talking about orbitals. So there's two different orbitals that can have these three quantum numbers, but if we're talking about electrons, we can also talk about m sub s, so if we have two orbitals, how many electrons can we have total? Yeah. So we have two orbitals, or four electrons that can have that set of quantum numbers.

So you'll notice in your problem-set, sometimes you're asked for a number of orbitals with a set of quantum numbers, sometimes you're asked for a number of electrons for a set of quantum numbers. So make sure first that you read the question carefully, and realize the difference that is between the two.

So, that's where we'll end today. So on Friday, we'll start with talking about the wave functions for the multi-electron atoms.