Lecture 29: Metals in Biology

Related Resources

Lecture Notes (PDF - 1.2MB) 

• Download this transcript - PDF (English - US)

The following content is provided under a Creative Commons license. Your support will help MIT OpenCourseWare continue to offer high quality educational resources for free. To make a donation or view additional materials from hundreds of MIT courses, visit MIT OpenCourseWare at ocw.mit.edu.

PROFESSOR: OK, let's just take 10 more seconds on the clicker question. OK, 76, I think that says, %, which is not bad, but we should be at 100%. So, when you're past the equivalence point, so you've converted all of your weak, in this case, acid to its conjugate base, and because it was a weak acid, the conjugate base is going to be a weak based and so it's not contributing a whole lot it'll make the solution basic, but it's nothing compared to adding strong base in there. So even though you have the weak base around, at this point it's really a strong base problem.

So you would calculate this by looking at how many mils of the strong base you've added past, and figure out the number of moles that there are, and divide by the total volume. So this was like one of the problems on the exam, and one thing that I thought was interesting on the exam is that more people seemed to get the hard problem right than this, which was the easy problem. So we'll see on the final, there will be an acid based titration problem on the final, at least one. So let's see if we can get, then, the easy and the hard ones right. So you've mastered the hard ones and let's see if you can learn how to do the easy ones as well for the final exam.

OK, so we're going to continue with transition metals. We were talking about crystal field theory and magnetism, and you should have a handout for today, and you should also have some equipment to make models of orbitals and coordination complexes -- these are not snacks. They can be snacks later, right now they're a model kit.

All right, so I'm going to introduce you to some terms that we're going to come back you at the end of today's lecture, and then we're going to talk about the shapes of coordination complexes. So, magnetism. So we talked last time, before the exam, if you remember, about high spin and low spin, unpaired electrons and paired electrons. Well, compounds that have unpaired electrons are paramagnetic, they're attracted by a magnetic field, and those where the electrons are paired are diamagnetic are repelled by a magnetic field. So you can tell whether a coordination complex is paramagnetic or diamagnetic, you can test the magnetism, and that'll give you some information about the electron configuration of the d orbitals in that coordination complex. And that can tell you about the geometry. And so you'll see that by the end we're going to talk about different types of energy orbitals when you have different geometries.

So why might you care about the geometry of a metal center. Well, people who study proteins that have metal centers care a lot about the geometry of them. So let me just give you one example. We talked a lot about energy in the course this semester, so we need catalysts for removing carbon monoxide and carbon dioxide from the environment. And nature has some of these -- they have metal cofactors and proteins that can do this, and people have been interested in mimicking that chemistry to remove these gases from the environment.

So let me tell you these enzymes are organisms. And this is pretty amazing, some of these microorganisms. So, over here there's one -- it basically lives on carbon monoxide. I mean that's -- you know alternative sources of energy are one thing, but that's really quite a crazy thing that this guy does. So, you can grow it up in these big vats and pump in carbon monoxide and it's like oh, food, and they grow and multiply, and they're very, very happy in this carbon monoxide environment. There are also microorganisms that live on carbon dioxide as their energy and a carbon source. And so these organisms have enzymes in them that have metal centers, and those metal centers are responsible for the ability of these organisms to live on these kind of bizarre greenhouse gases and pollutants.

So people would like to understand how this works. So microbes have been estimated to remove hundred, a million tons of carbon monoxide from the environment every year, producing about one trillion kilograms of acetate from these greenhouse gases. And so, what do these catalysts look like and these enzymes, what do these metal clusters look like that do this chemistry. And this was sort of a rough model of what they look like, and they thought it had iron and sulfur and then a nickel in some geometry, but they had no idea sort of where the nickel was and how it was coordinated.

And so before there was any kind of three dimensional information, they used spectroscopy, and they considered whether it was paramagnetic or diamagnetic to get a sense of what the geometry around the metal was. So we're going to talk about different coordination geometries and how many unpaired or paired electrons you would expect, depending on those geometries today.

And so, crystal field theory, again, can help you help explain/rationalize the properties of these transition metal complexes or coordination complexes.

So, to help us think about geometry, I always find for myself that it's helpful to have models. So not everyone can have such large models as these, but you can all have your own little models of these geometries. So, what we have available to you are some mini marshmallows, which, of course, as we all know, are representative of d orbitals, and jelly beans, which we all know are useful for making coordination complexes.

So, what you can do with your mini marshmallows is you can put together to make your different sets. And so, over here we have -- oh, actually it says gum drops -- you don't have gum drops this year, I changed up here, I forgot to change it down here. We have mini marshmallows. Dr. Taylor went out and tried to purchase enough gum drops to do this experiment, and discovered that Cambridge only had 300 gum drops, so we have mini marshmallows instead today. But this gives you the idea. You can take one toothpick and you can make d z squared, putting on your orbitals, you have your donut in the middle, and then your two lobes, which run along the z-axis. And then for your other sets of orbitals, you can take these two toothpicks and put on these sets of mini marshmallows, and handily, you can just have one for all of the other d orbitals, because depending on how you hold it, it can represent all of the other d orbitals just very well. So, you can just have one of these for all the others and then your d z squared.

So what we're going to do when we have our orbitals set up, then we can think about how ligands in particular positions, in particular geometries would clash with our orbitals -- where there'd be big repulsions or small repulsions. So, any other people missing their jelly beans or their marshmallows? Please, raise your hand, we have extras. So, those of you who have them, go ahead and start making your d orbitals.

All right, so if you're finished with your two d orbitals, you can start making an octahedral complex. So in your geometries set, you'll have a big gum which can be your center metal -- you'll have a big jelly bean -- sorry, big jelly beans and small jelly beans are our ligands, or our negative point charges, and you can set up and make an octahedral geometry here.

OK, so as you're finishing this up, I'm going to review what we talked about before the exam -- so this isn't in today's lecture handouts, it was in last time, which we already went over. But sometimes I've discovered that when there's an exam in the middle, there needs to be a bit of a refresher, it's hard to remember what happened before the exam, and you have your models to think about this.

So, before the exam, we had talked about the octahedral case, and how compared to a spherical situation where the ligands are everywhere distributed around the metals where all d orbitals would be affected/repulsed by the ligands in a symmetric fashion equally, when you have them put as particular positions in geometry, then they're going to affect the different d orbitals differently. And so, if you have your d z squared made, and you have your octahedral made, you can sort of hold these up and realize that you would have repulsion from your ligands along the z-axis directly toward your orbitals from d z squared. So that would be highly repulsive. The ligands are along the z-axis, the d orbitals are along the z-axis, so the ligands, the negative point charge ligands are going to be pointing right toward your orbitals. And if you hold up this as a d x squared y squared orbital where the orbitals are right along the x-axis and right along the y-axis and you hold that up, remember, your ligands are right along the x-axis and right along the y-axis. So, you should also have significant repulsion for d x squared minus y squared, and octahedrally oriented ligands.

In contrast, the ligands set that are 45 degrees off-axis, so d y z, d x z, and d x y, they're all 45 degrees off. Your ligands are along the axis, but your orbitals are 45 degrees off-axis. So if you look at that together, you'll see that whichever one you look at, the ligands are not going to be pointing directly toward those d orbitals. The orbitals are off-axis, ligands are on-axis. So there will be much smaller repulsions there. And that we talked about the fact that for d x squared minus y squared and d z squared, they're both have experienced large repulsions, they're both degenerate in energy, they go up in energy, whereas these three d orbitals, smaller repulsion, and they're also degenerate with respect to each other, and they're stabilized compared to these guys up here. So you can try to hold those up and convince yourself that that's true for the octahedral case.

So, that's what we talked about last time, and now we want to -- oh, and I'll just remind you we looked at these splitting diagrams as well. We looked at the average energy of the d orbitals -- d z squared and d x squared minus y squared go up in energy, and then the other three d orbitals go down in energy.

So now we want to consider what happens with different geometries. So now you can turn your octahedral case into a square planar case, and how am I going to do that? Yeah, so we can just take off the top and the bottom and we have our nice square planar case, and try to make a tetrahedral complex as well. And here's an example of a tetrahedral one. Again, you can take a jelly bean in the middle, and big jelly bean, and then the smaller ones on the outside. So what angles am I going for here in the tetrahedral case? 109 . 5. So you can go ahead and make your tetrahedral complex, and don't worry so much about the 0 . 5, but we'll see if people can do a good job with the 109.

OK, how are your tetrahedral complexes coming? Do they look like this sort of? So let me define for you how we're going to consider the tetrahedral case. So, in the tetrahedral case, we're going to have the x-axis comes out of the plane, the y-axis is this way, z-axis again, up and down. We're going to have one ligand coming out here, another going back, and then these two are pretty much in the plane of the screen. So this is sort of how I'm holding the tetrahedral complex with respect to the x, z, and y coordinate system.

So, there is a splitting, energy splitting, associated with tetrahedral, and it's going to be smaller than octahedral because none of these ligands will be pointing directly toward the orbitals. But let's consider which orbitals are going to be most affected by a tetrahedral case.

So, let's consider d z squared. What do you think? Is that going to be particularly -- are the ligands pointing toward d z squared? No. And d x squared minus y squared, we can think of, what about that one? No, not really. What about d x y, d y z, and d x y? Moreso. So, if you try holding up your tetrahedral in our coordinate system, and then hold your d orbitals 45 degrees off-axis, it's not perfect, they're not pointing directly toward them, but it's a little closer than for the d orbitals that are directly on-axis.

So, if we look at this, we see that the orbitals are going to be split in the exact opposite way of the octahedral system. In the octahedral system, the ligands are on-axis, so the orbitals that are on-axis, d x squared minus y squared and d z squared are going to be the most affected. But with tetrahedral, the ligands are off-axis, so the d orbitals that are also off-axis are going to be the most affected. But they're not going to be as dramatically affected, so the splitting is actually smaller in this case. So here, with tetrahedral, you have the opposite of the octahedral system. And you can keep these and try to convince yourself of that later if you have trouble visualizing it.

So, you'll have more repulsion between the ligands as negative point charges, and the d orbitals that are 45 degrees off-axis than you do with the two d orbitals that are on-axis.

So here, d x squared minus y squared and d z squared have the same energy with respect to each other, they're degenerate. And we have our d y z, x z, and x y have the same energy with respect to each other, they are also degenerate. So it's the same sets that are degenerate as with octahedral, but they're all affected differently.

So now let's look at the energy diagrams and compare the octahedral system with the tetrahedral system. Remember an octahedral, we had the two orbitals going up and three going down. The splitting, the energy difference between them was abbreviated. The octahedral crystal field splitting energy, with a little o for octahedral. We now have a t for tetrahedral, so we have a different name. And so here is now our tetrahedral set. You notice it's the opposite of octahedral, so the orbitals that were most destabilized in the octahedral case are now more stabilized down here, so we've moved down in energy. And the orbitals that are off-axis, 45 degrees off-axis, which were stabilized in the octahedral system, because none of ligands were pointing right toward them, now those ligands are a bit closer so they jump up in energy, and so we have this swap between the two.

So, we have some new labels as well. So, we had e g up here as an abbreviation for these sets of orbitals, and now that's just referred to as e. Notice the book in one place has an e 2, but uses e in all the other places, so just use e, the e 2 was a mistake in the book. And then we have t 2 g becomes t 2 up here. So we have this slightly different nomenclature and we have this flip in direction.

So, the other thing that is important to emphasize is that the tetrahedral splitting energy is smaller, because none of those ligands are pointing directly toward any of the d orbitals. So here there is a much larger difference, here there is a smaller difference, so that's why it's written much closer together, so that's smaller. And because of that, many tetrahedral complexes are high spin, and in this course, you can assume that they're all high spin. So that means there's a weak field, there's not a big energy difference between those orbital sets.

And again, we're going to -- since we're going to consider how much they go up and down in energy, the overall energy is maintained. So here we had two orbitals going up by 3/5, three orbitals going down by 2/5. So here, we have three orbitals going up, so they'll go up in energy by 2/5, two orbitals go down, so they'll be going down in energy by 3/5. So again, it's the opposite of the octahedral system. It's opposite pretty much in every way except that the splitting energy is much smaller, it's not as large for the tetrahedral complex.

All right, so let's look at an example, and we're going to consider a chromium, and like we did before, we have to first figure out the d count, so we have chromium plus 3. So what is our d count here? You know where chromium is, what its group number -- here is a periodic table. So what is the d count? 3. So we have 6 minus 3, 3 -- a d 3 system. And now, why don't you tell me how you would fill in those three electrons in a tetrahedral case. Have a clicker question there.

So, notice that in addition to having electron configurations that are different, the d orbitals are labelled differently.

OK, 10 more seconds. OK, very good, 80%. So, let's take a look at that. So down here, we're going to have then our d x squared minus y squared, d z squared orbitals up in the top, we have x y and x z and y z. Again, the orbitals that are on-axis are repelled a little less than the orbitals that are off-axis in a tetrahedral case. And then we put in our electrons, we start down here. And then one of the questions is do we keep down here and pair up or go up here, and the answer is that you would go up here. Does someone want to tell me why they think that's true? Yeah.

STUDENT: [INAUDIBLE]

PROFESSOR: Right, because it has a smaller splitting energy. So, the way that we were deciding before with the weak field and the strong field, if it's a weak field, it doesn't take much energy to put it up there. So you go they don't want to be paired, there's energy associated with pairing. But if there's a really huge splitting energy, then it takes less energy to pair them up before you go that big distance up there. But in tetrahedral cases, the splitting energy's always small, so you're just going to always fill them up singly to the fullest extent possible before you pair. So this is like a weak field case for the octahedral system, and all tetrahedral complexes are sort of the equivalent of the weak field, because the splitting energy is always small in an octahedral case, because none of the ligands' negative point charges are really pointing toward any of those orbitals that much, so it's not that big a difference.

So, here we have this and now we can practice writing our d to the n electron configuration. So what do I put here? What do I put first? So we put the e and then what? Yup. There are two electrons in the e set of orbitals, and in the t 2 orbitals, there's one. So that is our d n electron configuration. And then we're also asked how many unpaired electrons. Unpaired electrons and that is three.

All right. So that's not too bad, that's the tetrahedral case. The hardest part is probably making your tetrahedral complex. Now square planar. So again, with the square planar set you have your square planar model -- we have a bigger one down here. And the axes is defined such that we have ligands right along x -- one coming out at you and one going back, and also ligands right along the y-axis. So as defined then, we've gotten rid of our ligands along the z-axis. So, what do you predict? Which two of these will be the most destabilized now? What would be the most destabilized, what do you guess? You can hold up your little sets here. What's the most destabilized, what's going to go up the most in energy here? Yeah, d z squared minus y squared. What do you predict might be next, in terms of most unfavorable? Yeah, the x y one. So these two now are going to be the most destabilized, with d x squared minus y squared being a lot more destabilized than just the x y, because again, those d orbitals are on-axis and these ligands are on-axis.

So, let's take a look at all of these again. So in the octahedral case, these were degenerate. That's no longer true, because there are no ligands along the z-axis anymore. So we took those off in going from the octahedral to the square planar, so you have much less repulsion, but with the d x squared minus y squared, you still have a lot repulsion.

so then if we start building up our case, and this diagram is, I think, on the next page of your handout, but I'm going to start building it all up together. So now d x squared, y squared is really high up, it's very much more destabilized than anybody else. D z squared, on the other hand, is down. It's not -- it would be stabilized compared -- it's not nearly as destabilized as the other system.

So then we go back and look at these. You told me that d x y would probably be next, and that's a very good guess. You see you have more repulsion than in the other two, because the other orbitals have some z component in them. So you have less repulsion than d x squared minus y squared, because it's 45 degrees off, but still that one is probably going to be up a little bit more in energy than the other set. These two here are stabilized compared to the others, so they're somewhere down here. Now the exact sort of arrangement can vary a little bit, but the important points are that the d x squared minus y squared is the most destabilized, d x y would be next, and the other are much lower in energy. And we're not going to do this how much up and down thing, like the 3/5 and the 2/5 because it's more complicated in this case. So just the basic rationale you need to know here, not the exact energy differences in this particular case. OK, so now we've thought about three different kinds of geometries -- octahedral, tetrahedral, and the square planar. You should be able to rationalize, for any geometry that I give you, what would be true. If I tell you the geometry and how it compares with our frame, with our axis frame of where the z-axis is, you should be able to tell me which orbital sets would be the most destabilized. And to give you practice, why don't you try this one right here. So we have a square pyramidal case as drawn here with the axes labeled z, y and x, coming in and coming out. Tell me which of the following statements are true. And if you want, you can take your square planar and turn it into the geometry to help you out. Let's just take 10 more seconds.

All right. That was good. People did well on that question. So, if we consider that we had the top two are correct. So, if we consider the d z squared, now we've put a ligand along z, so that is going to cause that to be more destabilized for this geometry rather than square planar, which doesn't have anything in the z direction. ah And then in terms, also, other orbitals that have a component along z are going to be affected a little bit by that, but our other one here is not going to be true, so we just have all of the above is not correct, so we have this one. So if we had up those, that's actually a pretty good score.

And so you could think about, say, what would be true of a complex that was linear along z, what would be the most stabilized, for example. So these are the kinds of questions you can get, and I think there are a few on the problem-set.

All right, so let's come back together now and talk about magnetism again. So, we said in the beginning that magnetism can be used to figure out geometry in, say, a metal cluster in an enzyme, and let's give an example of how that could be true.

So, suppose you have a nickel plus 2 system, so that would be a d 8 system, so we have group 10 minus 2 or d 8, and it was found to be diamagnetic. And from that, we may be able to guess, using these kinds of diagrams, whether it has square planar geometry, tetrahedral geometry, or octahedral geometry. We can predict the geometry based on that information. Let's think about how that's true.

We have a d 8 system. Think about octahedral for a minute. Are there two options for how this might look in this case? Is there going to be a difference in electron configurations if it's a weak field or a strong field? So, write it out on your handout and tell me whether it would be true, think about it both ways. Is there a difference? So, you would end up getting the same thing in this particular case. So if it's a weak field and you put in 1, 2, 3, then jump up here, 4, 5, and then you have to come back, 6, 7, 8. Or you could pair up all the ones on the bottom first and then go up there, but you actually get the same result no matter which way you put them in, the diagram looks the same. So it doesn't matter in this case if it is a weak or strong field, you end up with those number of electrons with the exact same configuration.

So, we know what that looks like. Well, what about square planar. So let's put our electrons in there. We'll start at the bottom, we'll just put them in. I'm not going to worry too much about whether we can jump up or not, we'll just go and pair them up as we go down here, and then go up here, and now we've put in our eight electrons. So, how close these are, we're just going to put them all in. We're just going to be very careful not to bump up any electrons there unless we absolutely have to, because d x squared minus y squared is very much more destabilized in the square planar system, so we're going to want to pair all our electrons up in those lower energy orbitals. So even if we sort of did it a different way, that's what we would get. So we're going to want to pair everything up before we go up to that top one there. So there's our square planar.

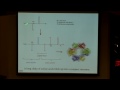
Well, what about tetrahedral. How are we going to fill these up? Do we want to pair first, or we do want to put them to the full extent possible singly? Single, right, it's going to be a weak field, there's not a big splitting here between these, so we'll put them in, there's 1, 2, 3, 4, 5, 6, 7, 8. All right, so now we can consider which of these will be paramagnetic and which will be diamagnetic. What's octahedral? It's paramagnetic, we have unpaired electrons. What about square planar? Square planar's diamagnetic. And what about tetrahedral? Paramagnetic. So, if the experimental data told us that a nickel center in an enzyme was diamagnetic, and we were trying to decide between those three geometries, it really seems like square planar is going to be our best guess. And so, let me show you an example of a square planar system. And so this particular nickel is in a square planar system. It has four ligands that are all in the same plane, and it is a square planar center for a nickel, so that's one example. And this is a cluster that's involved in life on carbon dioxide.

All right, so that's different geometries, you're set with that. Monday we're going to talk about colors of coordination complexes, which all have to do with the different geometries, paired and unpaired electrons, high field, low spin, strong field, weak field.

Have a nice weekend.

Free Downloads

Video

• iTunes U (MP4 - 92MB)
• Internet Archive (MP4 - 93MB)

Free Streaming

• VideoLectures.net

Subtitle

• English - US (SRT)