Lecture 28: Crystal Field Theory

Related Resources

Lecture Notes (PDF - 1.4MB) 

• Download this transcript - PDF (English - US)

The following content is provided under a Creative Commons license. Your support will help MIT OpenCourseWare continue to offer high quality educational resources for free. To make a donation or view additional materials from hundreds of MIT courses, visit MIT OpenCourseWare at ocw.mit.edu.

PROFESSOR: All right, so we started talking about transition metals, and at the end of last time we'd given an introduction to all the sort of nomenclature and things you need to know, and we had just gotten up to d orbitals, and when you're talking about transition metals you're talking about d orbitals. So, we're going to start with a little review of d orbitals, some of you have seen this before, maybe some of you have not. But here is what you're going to need to know about the d orbitals. You need to know the names of all the d orbitals, you should be able to draw the shapes of the d orbitals, and so the bar is not too high for this. You see the drawings that are in your handouts, you should be able to do about that well, it doesn't have to be super fancy. But you should be able to draw their shapes, and you should also be able to recognize which d orbital it is -- if you have a picture of different d orbitals, you should be able to name that d orbital.

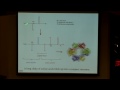
So let's just review what the d orbitals look like. And in this class, we're always going to have the same reference frame, so we're always going to have the z-axis up and down, the y-axis is horizontal, and the x-axis is coming out of the board and going into the board. So, we'll always use that same description. It'll often be given, but if it's not, you can assume that's what we're talking about.

So, the first d orbital we'll consider is d z squared. It has its maximum amplitude along the z-axis, and it also has a little donut in the x y plane. So, d x squared minus y squared has its maximum amplitude along the x and the y axis, so directly on-axis for this particular d orbital.

The next sets of d orbitals have their maximum amplitudes off-axis, so they don't correspond directly to the axes that I just mentioned, they're, in fact, 45 degrees off-axis. So we have the d y z shown here, d x z shown here, so maximum amplitude 45 degrees off z and x axes, and then z -- these pictures are a little bit complicated to see -- d x y, so it's 45 degrees off of the x and the y. And so, here I tried to draw them all in the same orientation of axes, which is a little bit difficult. So now let's look at them in terms of where they're drawn so you can kind of see them a little bit better, and so why don't you try to learn to recognize all of these. So, what is this one called? d z squared. What is this one, so its maximum amplitude is along x and y? d x squared minus y squared. I think this picture might be a little -- you might have somewhat aversion later on, but this is just good practice for you in recognizing them. So here we have one 45 degrees off-axis, which one is this? Yup. And this one over here? Yup. So, y z. And this last one here? Yup, we have the x z, so it's going up and down for the for z-axis.

So, a little more practice now. To show what these look like again, you want to think in three dimensions, and on paper and in most the time in Powerpoint you're not in three dimension, so here's a little movie in three dimensions. Here you can really see that donut, so this is d x squared -- see the maximum amplitude along z-axis here, and down here, and the little donut in the x y plane.

So this one is d x squared minus y squared. The maximum amplitudes are right directly along axis, so that allows you to distinguish it from d x y. So when it's on-axis here, it's the x squared minus y squared. So moving along here, so this is d x y, so you see that it's in that axis but it's not directly on-axis -- the maximum amplitude is 45 degrees off, so the orbitals are in between the axes there.

Now we're looking at one that has a z in it, and it looks like it's x z, so that's where our maximum amplitude is between the x and the z-axes, 45 degrees off.

And our last one, we have y and z here. Again, 45 degrees off-axis between the y-axis and z-axis.

So, hopefully these little movies will help cement in your brain, what the shapes of these d orbitals are.

All right, so that's d orbitals, and we're going to be mentioning d orbitals in every lecture in this, and you have to be thinking about what the shapes of the d orbitals are to talk about today's topic, which is crystal field theory. So, there are two types of theories that you may hear of and that your book mentions -- crystal field theory, and ligand field theory, and like most things that you learn about in freshman chemistry, the theories were developed to explain experimental information. So there are special properties of coordination complexes, so that's where you have a transition metal in the middle and you have ligands all around it, so you have these coordination complexes and they have special properties. And so people wanted to try to rationalize these special properties and they came up with these two theories.

So, the basic idea behind these theories is that when you place a metal ion with the particular oxidation number in the center of a coordination sphere, and you have all these ligands, these donor ligands, all surrounding them, that the energy of the d orbitals is going to be altered by the position of those ligands. So it's all about the d orbitals, and the d orbitals are going to experience some influence from these ligands, these donor ligands that are surrounding the metal. So then, between these two theories that are used to explain how these d orbitals are being affected.

The crystal field theory is based on an ionic description, so it considers the ligands as negative point charges. It's a very simplified model, whereas as the ligand field theory considers covalent, as well as ionic aspects of coordination. It's more powerful it's more useful, but it's also a bit more complex, and so we don't cover it in this of course, and if you go on and take the first level of inorganic chemistry, which is 503, then you'll hear about this. But for this course, we're just going to talk about crystal field theory. Even though it's very much of a simplified model, it actually works very well. You can explain quite a few properties of coordination complexes just using this simplified method.

So, crystal field theory, again, very simple. It's just considering the ionic interactions, it considers the ligands as negative point charges. And so, the basic idea is that ligands, as negative point charges, are going to have repulsive effects if they get close to the d orbitals. So here is a drawing of a metal, and so this is metal abbreviated m, its oxidation number is m plus here, and it has ligands all around it. What is the geometry here? It's octahedral geometry. And so we have ligands up and down along z, ligands along y, and a ligand going back along x, and a ligand coming out along x. And so here's another picture of the same thing, the metal is in the middle, and the ligands -- in this case, you have these ammonia ligands or these little negative point charges, which are all along the axes. You have four along the equatorial, and one up and one down, so this is the octahedral geometry. And so you can just think about each of these ligands as negative point charges. And so, if the negative point charge is pointing right toward a d orbital, that'll be very repulsive. If it's not it's less repulsive. That's the whole idea behind this crystal field theory.

So, here again, is just another little picture, so you can kind of get the idea that we're going to be thinking about the all the shapes of the d orbitals, and we're going to think about where the ligands are. Today we're going to talk about octahedral geometry, but we're also going to go on and talk about tetrahedral geometry later. So here in octahedral geometry, you can think about the positions of all of these negative point charges surrounding your d orbitals. And when the d orbitals are on axis, like the ligands, there's going to be more repulsion, so you can see here that would be quite repulsive -- you have a negative point charge by that d orbital. When the d orbitals are off-axis and the ligands are on-axis, that's less repulsive. And that's the basic idea.

So, let's look at each one of these orbitals now in detail and think about how a ligands that's pointing directly toward it is going to be affected. So we have the ligands, l, as these point charges directed toward the d z squared, and the d x squared minus y squared orbitals, and these would result in quite a bit of repulsion. So if you had a ligand right up here along z, and so that would be a very close interaction. In this case, you're going to have ligands along x and y, again pointing directly toward the orbitals, that would be quite repulsive.

And I'll just mention, we'll come back to this later, that one can think about the case where you have the octahedral geometry where the ligands are in a definite position, and you can also think about this sort of hypothetical case where you have a metal in the middle and you have the ligands, here are the little ligands, and they're everywhere, there's ligands everywhere all around. And so, in this case where you have ligands everywhere all around your metal, then all your d orbitals would have the same energy, but if you take the ligands and you isolate them in particular positions, then you can consider how the different shapes of the d orbitals will be affected. We'll come back to that in a minute.

All right, so here we have a case where our ligands are on-axis, our orbitals on-axis, this is a large repulsion. So, I will tell you that d x squared and d z squared and d x squared minus y squared orbitals are destabilized, and they are destabilized by the same amount. So there's repulsion now, and so they're destabilized, and they're destabilized by the same amount of energy.

So what's it called when orbitals are of the same energy? Yup. So, d z squared and d x squared minus y squared are degenerate. So, d z squared and d x squared minus y squared orbitals are destabilized more than the other three orbitals, and let's consider now why that is true.

So here are our other sets of orbitals, and remember, here the maximum amplitude of these orbitals are 45 degrees off-axis, whereas our ligands are all on-axis. So, the ligand negative charges are directed in between these orbitals, not directly toward them. So that is stabilized compared to this hypothetical case where the ligands are everywhere, so some of them will be pointing toward them, and also stabilized compared to the other sets of orbitals where the ligands are now pointing directly at them.

So these three sets of orbitals are stabilized relative to the d z squared and the d x squared minus y squared orbitals, and they're stabilized by the same amount. So these three orbitals are also degenerate with respect to each other.

So then to sort of summarize this set of orbitals, we have for d z squared and d x squared minus y squared, we have large repulsions by those negative point charges, they're pointing directly at the orbitals, and so they're destabilized, higher in energy than the other -- the d x y, d y z and d x z. For the d y z, d x z and d x y, they're smaller repulsion, because these orbitals are off-axis, and so the negative point charges aren't pointing directly at them. So they're stabilized relative to these guys up here.

So that's the whole idea behind an octahedral case of crystal field theory. And we can look at this just one other way, if pictures help you. Here it's a little clearer that those negative point charges are pointing directly toward the orbitals, here I think you can see that the negative point charges are not directly pointing toward any of the orbitals. So I'll show you a bunch of different figures, this all shows you the same thing, but some might help you see this relationship better.

OK, so now we're going to draw some diagrams. I'm going to start over here. So we're going to draw what's called a crystal field splitting diagram, and this is for an octahedral case. And the diagrams are going to look different depending on what the geometry is. So when we draw this diagram, energy is going up, and we're going to start with our 5 d orbitals, and so this is going to be the average energy, the average energy of our d orbitals. And so, this is then with a spherical crystal field. So that's where the ligands are distributed around uniformly. So it's all spherical, they aren't set up in the octahedral case yet, our octahedral diagram's going to be over here, but this is the case that this represents. If you have all your ligands spherically distributed around your metal, then the energy of all the d orbitals are identical, because every d orbital has the same amount of ligands, it's uniform, it's symmetrical all around the metal.

And I just want to tell you that this is was very exciting to me when I saw this. I've been teaching this class for a while, and I never had a real spherical crystal field around my metal before. And then I walked into Walgreens one day, and I was very excited to see that Walgreens sold spherical crystal fields. I mean you never know what you're going to get. I'm a big fan of Walgreens, I've found a lot of good stuff, toys for my dog, etcetera, but this was really amazing. So I asked the cashier on the way out whether they knew they were selling spherical crystal fields, and they did not actually. So, you just never know what you're going to get.

OK, so in that case, where the ligands are uniform all around, the energy is the same. But now, if we have an octahedral crystal field over here, so we have our octahedral crystal field, then we get some splitting. So some of our orbitals are going to be destabilized, and they'll be higher in energy here. So we have the d x squared minus y squared, and d z squared over here are going to be higher in energy. And we're going to have three that lower in energy, so we'll have our d x y, our d y z, and our d x z over here, will be lower in energy. This difference is called the octahedral field splitting energy, because it's the amount of energy that the octahedral field is split.

So over here, we can put this is for the octahedral case, crystal field splitting energy. And again, some of the orbitals go up in energy, some of the orbitals go down in energy, and the overall energy needs to be conserved. So, if two orbitals go up in energy, and three go down in energy, then to have everything add up, you can say that three go up in energy by 3/5, and two, these three orbitals are going to go down by 2/5. So overall, the energy of the system is maintained.

OK, so that's a crystal field splitting diagram for an octahedral case, and now let's look at some examples of this. So let's look at an example, and we're going to have a chromium system that has three n h 3 ligands and three b r ligands. Now, you tell me what the d count of that is. Let's just take 10 more seconds. They're not as high overall, but still more people got the right answer.

So, let's take a look at this. What's the oxidation number of bromium? What is it? Bromium? What's 1 b r minus? What's its oxidation number. Minus 1. There are three of them. What about ammonia? 0. So, 3 times 0. And the overall charge of this is 0, so there's nothing up there. So what does that mean about chromium? Plus 3.

All right, so now we have to figure out the d count. So the d count is going to equal the -- and the periodic table, the group number, so if you switch to my slides, we can see what that is. So what is that for chromium? 6. And then we have 6 minus 3, because our oxidation number is 3, and so we have a d 3 system. So, did some of you get this wrong because you stopped too early? Here, the answer, if you had 3, you could have stopped with oxidation number and still gotten it correct. So that's a d 3 system. So, we're going to worry about three d electrons, and we're going to put three d electrons into our splitting diagram.

OK, so if you had a hypothetical spherical crystal field, you would have your one's in here, but now let's consider what happens in the octahedral case. So we can come down here. Am I going to put my first electron down here or up here? Down. Oh, I just realized that I didn't put two things on this diagram, so these are in your notes, but these diagrams have little abbreviations in them for the orbital levels. So we have an e g and t 2 g, and that's an abbreviation for the names of the ts of orbitals, which you'll see later is very convenient in terms of writing things out.

All right, so we're going to put them down here. Am I going to put two of them together with the spins up in the first orbital? No. So you know that that is not good, give you the same four quantum numbers, you don't want to do that. So, we can put them in. What about just putting in a paired set over here yet? No. They have the same energies here, so we're going to put them in all singly, and then we're done. So we put three electrons in these three orbitals.

Now we can introduce a couple other terms, which is where I realized I forgot to put the labels on. So, you'll often be asked for -- OK, you'll often be asked for something called the d n electron configuration. And so here you can use the abbreviations for the orbitals, so we point three electrons in to the t 2 g orbitals, so we can just say that's t 2 g raised to the three. So that let's someone know that you have three electron in the set of orbitals that are stabilized in an octahedral crystal field. Then we can consider something else that's called c f s e, and I think I should have -- OK, so I'll write that out. So, this is the crystal field stabilization energy. So it's not the crystal field splitting energy, it's the stabilization energy, which indicates how much those electrons are stabilized by being in an octahedral field, rather than this hypothetical spherical crystal field. And so what we can do there is you see that you have three electrons in those lower sets of orbitals, and those orbitals are stabilized by 2/5 times the octahedral crystal field splitting energy. And so that gives an answer of minus 6/5 times the octahedral crystal field splitting energy. So that's how much those electrons are stabilized. So they are lower in energy -- see, the average energy is much, much higher in this hypothetical case, but because of having this octahedral geometry, and there are only three electrons to consider, they all go into the stabilized energy, and so they're stabilized by minus 6/5 times whatever the octahedral crystal field splitting energy is for this particular case.

So, now let's look at another example. So let's look at an example of a coordination complex -- you have manganese and you have six water ligands and some chlorides hanging around. So why don't you tell me what the oxidation number is for this now -- not the d count, but the oxidation number? OK, so let's just take 10 more seconds. OK, so let's just look at that one for a minute, people did very well on that.

So, what's the overall charge in this coordination complex? So we can write this out here. Our six ligands and we say that here it's plus 3 overall, because we have three chloride ions hanging around with a negative charge. So this tells us that the overall charge on the coordination complex had to be plus 3. And this again is 0, so that means that is plus 3. So, people did very well on that.

All right, so then what is the d count? What is it? 4, right. So we have 7 minus 3 is 4, so we have a d 4 system.

All right, so now, if you look up there, we have to make a decision about that fourth electron. three electrons were easy, four makes it complicated. Do we put the fourth one down in the lower set or do we go up? So there are two possibilities here. So I have two diagrams drawn over here. And you might be in a case where you have a small crystal field splitting energy, or you might be in a case where you have a large crystal field splitting energy. And so, there are two different ways the electrons can go.

So over here where you have a small crystal field splitting energy, that's called a weak field. And when you have a big splitting energy, that's a strong field. So, with the weak field there's not that much of an energy difference between them. And so, when you're putting in, you do your first three electrons, that's always going to be the same. But then the fourth electron, in this case, if there's not a big difference in the energy, if it's pretty small, if it's a weak field, you can put that fourth one up there. Because it takes energy to pair the electrons up, and so you're asking the question, does it take more energy to pair them, or does it take more energy to put one in the upper set? And for a weak field you say that the crystal field splitting energy is smaller than the pairing energy or p e, so p e is the pairing energy. And so it takes more energy to pair than it does to bump one electron up to the higher level. So that's what a weak field situation would look like.

Now in a strong field situation, boy, there's a big splitting difference, a big energy difference here. So in this case, the crystal field splitting is much larger than p e, that pairing energy for the electron. So it's better to pair, then to put one up. I can't even reach those, that's really far up. So I'm not going to do that, I'm just going to put them in here. I'll put the first three in, and then the fourth one is going to go down where I can reach it. I don't have the energy to put it up there. So that's a strong field. Weak field I can handle, strong field I'm going to try pair them all up.

So, now we can write the different d n electron configurations for these two. So, in this case, if we have our d n electron configuration, so we have three in the t 2 g, we have three. And in the e g set we have one, so that is our electron configuration for this weak field case. And for the strong field case, we didn't put any up in the e g, so we just have t 2 g, four electrons in that set of orbitals.

OK, so let's just put up what we've done here, and introduce another term, which are high spin and low spin. So we have these two cases here, and again, we're considering how big is this octahedral crystal field splitting energy compared to a pairing energy. The energy involved in pairing electrons together. In a weak field, the splitting energy is small, so electrons are placed singly with spins parallel to the fullest extent in all the sets of orbitals. In this other case with the strong field, the pairing energy is smaller than the splitting energy -- strong field you have a big splitting energy. And so, in that case, with the splitting energy is large, you're going to put all your electrons in and pair them up in t 2 g and don't put any electrons in the e g sets of orbitals until you completely filled your t 2 g set. So, the net result of this is for a weak field, you have the maximum number of unpaired electrons, so see, you have a maximum number, you have four electrons, all four are unpaired. So that's the maximum number of unpaired electrons, and this is referred to as high spin. And in the other case, you have the minimum number of unpaired electrons that you can have, and so you have this one set that's paired here, so that would be considered a low spin case.

We can also talk about the stabilization energy of these two cases, and so we have the crystal field stabilization energy. So why don't you go ahead and tell me for a weak field case, what is our stabilization energy? Let's just take 10 more seconds. OK, pretty good. So let's work this out.

So, first we consider how many electrons we have in the lower set of orbitals, so we have three. Those three are stabilized by 2/5. And then we have one in the upper set, so we have one at 3/5 times the octahedral crystal field splitting energy. So this ends up with minus 3/5 times the octahedral crystal field splitting energy. And notice why a is not correct -- you don't have the symbol for the octahedral crystal field splitting energy. On a test, you need to make sure that you remember to write this term, so this was a little test so that you hopefully emphasize that you want to have that there.

All right, so that's for this one. Let's look at the low spin case, the strong field case, and do our crystal field splitting energy for that. So in this case we're going to have four electrons times minus 2/5 times the octahedral crystal field splitting energy, so that's equal to minus 8/5 times the octahedral crystal field splitting energy, and some books will also indicate, and they'll say plus p e to indicate that there's pairing energy that's associated there. So it's not quite as beneficial as one might think. You do have a lot of electrons in these in lower energy orbitals, but you did have to pair some of them, so you had one, and if two of them are paired, you can see sometimes 2 p e. So, some books will do that, some books will not. So I just wanted to mention that both are OK. And whether you're asked to write that or not, and the question will say include the pairing energy, so you know whether you're supposed to do that or not, that there is that energy associated with pairing in this strong field case.

OK, so let's look at another example, and then you should be all set to do these problems for an octahedral field. So we'll take our electrons down. All right, so let's at a case of cobalt plus 2. So let's consider how many we're going to put in, the oxidation number is? Plus 2. And what is the d count then? What group number? 9 minus 2 is 7, so we're doing a d 7 system.

All right, so now for the weak field case here, why don't you tell me what is the correct electron distribution. OK, let's just take 10 more seconds. OK, very good. This is the weak field case, so in this case, we are going to fill up singly all of the orbitals, because the splitting energy is less than the pairing energy, so we're going to put them to the fullest extent possible before we have to pair. So we have 7, so we're going to do 1, 2, 3, 4, 5, and now we have to pair. We have no -- we've used up all our orbitals, so we're going to start pairing down here in the lower energy, so that would be 6, 7. So we had no choice, we had to pair them, but because it was a weak field, we filled them all up singly first before we paired.

So now let's consider what we're going to do down here. Now remember, this is a strong field, so there's a big splitting energy, so it takes less energy to pair them than to reach this higher level. So first we put them in the same way -- 1, 2, 3, but now that we have the 3 in, it's better to pair than to bring them up here. So we'll do 1, 2, 3, we'll pair them all up. Now it's all filled, we have no other choice but to go up here.

So, we have these very different cases depending on the splitting energy. So what controls the splitting energy? Well, what controls the splitting energy is nature of the ligands, so we're going to be talking about that next time, and you'll recognize for certain kinds of ligands you're going to have a strong field, and other kinds you'll have a weak field. But right now we're not talking about that, we're just showing those two possibilities.

So, for this system, is this going to be a high spin or a low spin system? So, this will be high spin, because we have the maximum amount of unpaired electrons. And over here we're going to have a low spin system. We have the minimum number possible of the unpaired electrons.

All right, so let's finish this up now, and do our d n electron configurations and our crystal field splitting energies. So, for this case, we're going to have in our t 2 g system, how many? 5. And in e g? 2. And for our splitting energy, we have 5 times minus 2/5 times the octahedral crystal field splitting energy plus 2 times plus 3/5, and what does that end up equaling? Minus 4/5 times the octahedral crystal field splitting energy. And we could also optionally put 2 p e because we have two sets paired. All right, so let's look at our strong field system. How many do we have in our e 2 g set? 6. What about e g? 1. And for our splitting energy then, we have 6 times minus 2/5 times the octahedral crystal field splitting energy plus 1 times 3/5, and what is that going to equal? Minus what? 9/5. And how many pairing energies? three pairing energies, great.

I think you have that part down. Next time we get more complicated, we're going to talk about types of ligands, we're going to talk about tetrahedral, we're going to talk about square planar, and that's, of course, after the exam on Wednesday. So, good luck, everyone, with the exam on Wednesday.

Free Downloads

Video

• iTunes U (MP4 - 101MB)
• Internet Archive (MP4 - 100MB)

Free Streaming

• VideoLectures.net

Subtitle

• English - US (SRT)