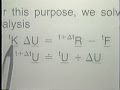Lecture 5: Updated Lagrangian Formulation - Incremental Analysis

Related Resources

Study Guide (PDF)

Readings

Sections 6.2.3, 6.2.4

References

Bathe, K. J., E. Ramm, and E. L. Wilson. “Finite Element Formulations for Large Deformation Dynamic Analysis.” International Journal for Numerical Methods in Engineering 9 (1975): 353-386.

Bathe, K. J., and H. Ozdemir. “Elastic-Plastic Large Deformation Static and Dynamic Analysis.” Computers & Structures 6 (April 1976): 81-92.

• Download this transcript - PDF (English - US)

The following content is provided under a Creative Commons license. Your support will help MIT OpenCourseWare continue to offer high quality educational resources for free. To make a donation or view additional materials from hundreds of MIT courses, visit MIT OpenCourseWare at ocw.mit.edu.

PROFESSOR: Ladies and gentlemen, welcome to this lecture on nonlinear finite element analysis of solids and structures. In this lecture I would like to discuss with you the updated Lagrangian formulation for general incremental nonlinear analysis.

However, before doing so, I thought it would be good to take a bit of time and review some of the material that we have been discussing already. We have been encountering a large number of quite complicated equations, some very important basic concepts, and some subtleties. And to really understand all of this material, it is necessary to study it, of course, a lot more than the time that we have allocated so far. But it might help you to just review now some of that material once more.

I've prepared here on the marker board the material that relates to the total Lagrangian formulation, in which really you have been seeing some of the very important equations. And here we have the basic equation that is used, of course, in finite element analysis. This basic equation states, of course, it is the equation of the principle of virtual work, states that an internal virtual work, or the internal virtual work, must be equal to external virtual work.

You remember that we talked about the Cauchy stress, the stress which is actually force per unit area. And that's the engineering stress, that's the stress that we want to solve for. That we talked about a virtual strain. A virtual strain that is referred to time t plus delta t. And that is why we have this subscript here, t plus delta t. And we integrate this product here over the volume at time t plus delta t. Notice that I'm using here a green color for the real stress that we want to solve for, and a red color for the virtual quantity that we're using basically as a mechanism to solve for this stress.

Of course, on the right-hand side, we identified we have the external virtual work. And in here, this script R here, denotes contributions from the surface forces, body forces that are being applied externally to the body. This is really the basic equation that we want to operate on, and that we want to work with to solve for the unknown Cauchy stresses at time t plus delta t. Now we recall, of course, that we are here integrating over a volume at time t plus delta t, and that volume is unknown.

Because it is unknown, we cannot very easily deal with this stress measure, and we have, therefore, introduced a new stress measure. A stress measure that we call the second Piola-Kirchhoff stress tensor. We introduce this one here. Notice this is a stress at time t plus delta t, referred to the original configuration, 0, of the body. The capital S, and we have ij as the components of that stress tensor.

Now, this is a new stress tensor that we know we can deal very well with. And we have to also introduce a new strain tensor. Why do we have to do so? Well because using this stress tensor, we have to use an energy conjugate strain measure. And this Green-Lagrange strain tensor, the Green-Lagrange strain tensor is energy conjugate to the second Piola-Kirchhoff stress tensor.

Having introduced these two quantities, and we looked at the components, how they're constructed, how they're related, for example, in this case, how this one is related to the Cauchy stress. Having now introduced these two quantities, we can directly work basically with this equation here. Well to do so, we have decomposed the stress tensor into a part that we know, and into an unknown part.

And that's an important point. We can decompose this stress tensor into a stress that we know, and that we don't know, by simple addition here on this side. Notice we simply add here because we had a quantity that is referred to the volume at time 0, to another quantity that is referred to the volume at time 0.

In other words, the reference configurations for both of these are the same, and that's why we can have a simple addition sign here. Please keep in mind that this quantity is known in our incremental solution. Because we, of course, assume that we have solved for the configuration of the body up to a time t. This one is unknown, and this is the reason why I used here black color to show that this is an unknown quantity and distinguish it from the one that is known.

We proceed in the same way with the Green-Lagrange strain tensor. Here we have the equations. The Green-Lagrange strain tensor at time t plus delta t is decomposed into a quantity that we know, plus an unknown quantity. Once again, in black. So these are the two quantities that we really want to solve for, the increment in the second Piola-Kirchhoff stress, and the increment in the Green-Lagrange strain.

Now we also discussed that the increment in the Green-Lagrange strain can actually be decomposed into two quantities. One that is linear in the particle incremental displacements ui, and one that is nonlinear in the particle incremental displacements ui. Please read it as follows. Linear in ui, nonlinear in ui.

Of course, remember that we have so far only spoken about the continuum mechanics. In other words, we have not really, at this point, said anything about the finite element discretization. We also referred, however, in an earlier lecture to the fact that if we do interpolate in finite element analysis, then using continuum elements for the analysis of solids and structures, the incremental nodal point variables are linearly related to these incremental particle displacements. And for that reason, this is a true linear strain increment, and that is a true nonlinear strain increment.

On the other hand, if we use structural elements that also include or use nodal point rotations, then the particle displacements, these ui's, are linearly related to the incremental nodal point displacements, but nonlinearly related to the incremental nodal point rotations. And in that case, this is here still the true linear increment of the Green-Lagrange strain for the particle. But this one here is not containing all the nonlinear strain incremental parts for the particle. There has to be a little bit-- there has to be some amendment here, some addition here. And we briefly talked about the effect of neglecting this addition as well.

Well the point now is that we want to use, of course, these two quantities to substitute these into the equation of the principle of virtual work, and we then arrive directly at this relationship here. Now what do we see here? We see an integral over the original volume of the second Piola-Kirchhoff stress referred to the original configuration, and it's a real stress, the one that we want to solve for, and that's why I use the color green again.

This here is a virtual strain, Green-Lagrange strain. It's a variation on this Green-Lagrange strain here. And this product is integrated over the original volume, which is, of course, the volume that we know. And this is the reason why we can so well deal with this integral.

On the right-hand side, we have made no modification. We still have simply the external virtual work. Now, please realize that this equation here is totally equivalent to the equation that we discussed earlier. This equation here. Here we are talking about Cauchy stresses, an infinitesimal virtual strain integrated over a volume of time t plus delta t that is unknown. This equation has simply been re-cast into a new form using continuum mechanics. No finite elements really, just continuum mechanics, into this form here.

And now we have a form that we can work very well with. We substitute into this equation and we linearize, and we did that in the earlier lecture. The result of that substitution and linearization is given right here. Now notice that in each of these integrals, of course, we are integrating over a volume that is known, the original volume. Notice that in the first integral here, we involve, well a material tensor. That has to be defined, it depends on the material that you're looking at, that you want to analyze.

This is here the increment in the Green-Lagrange strain, the linear part only. Here is a variation on this linear increment in green-- sorry, it's in red, of course. This one was green. This one is in red. And this is, of course, a virtual quantity. This is the virtual quantity. Notice that here we are talking about a stress that we know. We're incrementally decomposing. Remember? We're adding to the quantities at time t, the increment. The quantities at time t are known. So this one is known.

This one here is a variation on the nonlinear increment of the Green-Lagrange strain, total increment. Well, in red again. And on the right-hand side we have the external virtual work, which of course, is assumed to be given. Do you still remember that we are talking about deformation independent loading. So we can calculate this piece here directly. And here we have also the stress that is known, the stress at time t, and the virtual strain, linear strain.

This is what we arrived at in our earlier lecture. Of course, there was a linearization involved, and we know there would be errors if we simply operate on this equation. We don't want to have these errors in the actual finite element solution. And we realize that it is good to iterate. So we also looked in the earlier lecture how we would iterate typically. And we developed an equation where there was something on the left-hand side, which I simply denote here by dots.

There were quite some complicated expressions. You may want to look them up again. But I just denote them by dots because what is really important, and that I pointed out also earlier, is what is on the right-hand side.

And what do we see on the right-hand side? On the right-hand side we see the external virtual work, and we are subtracting from it this integral. Let's look at this integral once more. We have here the second Piola-Kirchhoff stress at time t plus delta t. Of course, always refer to the original configuration. Corresponding to iteration k minus 1. The iteration here written in black.

We are operating on the Green-Lagrange strain at time t plus delta t. Refer to the original configuration in iteration k minus 1. Actually, calculated at the end of iteration k minus 1 is better to say. And this product here is integrated over the original volume once more. k runs from 1, 2, 3, onwards.

Well notice that when k is equal to 1 we have a 0 here, and we have to ask ourselves what is this quantity then? Well if there's a 0 right here, then we're looking here at t 0 Sij. The stress, the second Piola-Kirchhoff stress corresponding to the configuration at time t. And if we put a 0 here, we would have also here, the initial condition being the Green-Lagrange strain at time t, of course, referred to configuration 0. So we have the write initial conditions here, and therefore we can proceed with the iteration. We discussed that in the earlier lecture.

The important point now is that once this iteration converges, we have to use, of course, schemes, solution procedures that makes the iteration converge. We will talk about that in later lecture. But once we have obtained convergence, and let's assume we have converged, then this R, the script R, the external virtual work, is equal to this integral. That's how we define convergence. And this means then that the external virtual work is now equal to the internal virtual work.

What does this mean? It means in essence, that this right-hand side is 0, which means-- and now I like to go back to our earlier equation-- that this equation here is satisfied because notice that if you look at this equation and you take this left-hand side and bring it to the right-hand side with a minus in front, then you have exactly what we just looked at earlier.

In other words, our iteration is performed really on an expression that is nothing else than this R, this script R, minus this one on the right-hand side. There's a minus sign here and you take this and bring it right there. That's what we just looked at.

Of course, we also remember now that this expression now is really totally equivalent to what we started with. Namely, this expression right here. And this is, of course, what we really want a solve. We mentioned earlier that we introduced the second Piola-Kirchhoff stress, and that we would like to get a physical understanding possibly of that stress. And I mentioned that that is very difficult to obtain.

In the computer program, we just work with that stress measure in order to be able to solve the equation, to operate well on the principle of virtual work. What we really want to do, of course, is to solve this equation right here. This is the equation. That is the physical stress that we want to solve for.

Well since this equation is totally equivalent to that equation, and looking at this equation, if we take this left-hand side, bring it to the right-hand side-- of course, there's a minus appearing there-- we do obtain what we see right here at convergence of course. We operate properly on the principle of virtual work.

So this really closes, so to say, the loop, and I hope it helps you to have this overview in understanding of what I discussed earlier with you. But let's go one step further. In the finite element discretization, we obtain from this equation here-- I haven't listed what is on the left-hand side, you can look it up-- we obtain directly this equation here. Of course, there's quite a number of steps that we still have to discuss.

How we obtain this matrix, we call this the tangent stiffness matrix. We have to discuss how we calculate this vector here, this vector here. In fact, the way we actually introduced it, since it's a total Lagrangian formulation, I should really put here a 0. We have to discuss how we can calculate this vector for different elements, et cetera, and there is a lot still to be learned.

But remember that up to here, we only talked about continuum mechanics, and this is where the finite element discretization starts. And these are then the basic equations that are being arrived at. At convergence, once again, here we iterate, of course. Notice that I'm using now the superscript i. Of course, this superscript is a dummy, it could be k, it could be j, it doesn't matter. We frequently use i. I didn't want to use up here i because we have an i already as a subscript, that's why I'm using k here.

But at convergence in this iteration where we calculate always new displacements, add them to the previously calculated displacements, at convergence the right-hand side will be 0, or close to 0, of course.

Well this means that we arrive at this equation right here. Because when the right-hand side is 0, we satisfy that t plus delta t R is equal to t plus delta t F And if you like, here also, we may want to introduce this little 0, signifying that we have used the total Lagrangian formulation to calculate that F.

Now it is important to realize what we have achieved in the finite element solution. We satisfy compatibility in the finite element solution if we use compatible finite elements. That is buried in here. We use compatible finite elements to calculate this F, therefore we satisfy compatibility. We satisfy the stress strain law properly if we calculate this F here accurately and properly through the stress strain law. Of course, there is much to be discussed still. But let's assume we do that properly and then we satisfy the stress strain law.

Equilibrium we also satisfy, but at the nodal points. We talked about the fact, in an earlier lecture, that in a finite element analysis, displacement based finite element analysis, we always satisfy equilibrium at the nodal point. Of course, assuming that the elements have been properly formulated. We would only satisfy local equilibrium if the mesh is fine enough. And we talked earlier, in an earlier lecture, about how we can measure whether local equilibrium is well enough satisfied using stress jumps, using pressure band plots. We talked about that and I gave you some examples.

The important point though is to recognize that if we use a fine enough mesh, then equilibrium is satisfied, and the stress strain law would be satisfied if we calculated stresses properly from the given strains. And compatibility is satisfied if we use a compatible finite element mesh. In other words, the three basic important requirements of mechanics are fulfilled when we are satisfying this relationship right here.

This basically then rounds up what we have been discussing so far. And we use the total Lagrangian formulation in this discussion. I now like to go over to discuss this, use the updated Lagrangian formulation. The interesting point here is that we use a stress measure that is referred to the original configuration, and so a strain measure that is referred to the original configuration.

I mentioned earlier already that we want to deal with a certain configuration that is known. The reason, of course, being that we want to incrementally decompose this stress into a quantity that we know, plus a quantity that we don't know. And that incremental decomposition should involve stress measures that are referred to a configuration that is known. The same for the strains.

Well since we have calculated all the configurations from time 0 to time t already, a natural question to ask is why don't you use a configuration other than 0, say, the configuration at time delta t to delta t, or t. well in the updated Lagrangian formulation, we, indeed, use the configuration at time t as a reference configuration. And so we deal then with the second Piola-Kirchhoff stress referred to the configuration at time t.

This is the stress that we're dealing with. Notice t plus delta t here, still the stress at time t plus delta t, but now the stress is referred to the configuration at time t. Similarly, we also deal with a strain.

The Green-Lagrange strain at time t plus delta t, but referred to configuration at time t. We could, of course, ask, well why use a configuration at time t? Why not use some configuration in between? The answer is, and I gave it to you already in an earlier lecture, the answer is that you are losing all of the advantages of the total Lagrangian formulation, and you're losing the advantages of the updated Lagrangian formulation. You are left only with the disadvantages. So there's very little point really in trying to solve the principle of virtual work using another configuration other than 0 or t.

Let us now look at the details of the updated Lagrangian formulation. And here I have now prepared some view graphs that show all the equations that we're operating on.

The principle of virtual work, once again, is given right on this first view graph. We always start with the basic principle of virtual work, and that is written here. This is, once again, the Cauchy stress, the stress that we are actually interested in solving for. This is the infinitesimal virtual strain. Internal virtual work is equal to external virtual work.

Now, with the stress measure that I just mentioned to you, and the corresponding strain measure, substituted for these stress and strain measures, we obtain directly this equation. Notice that here on the left-hand side, we have an integral that is exactly equal to this integral here. And that we are not talking here about finite elements yet. It's all continuum mechanics at this point.

The second Piola-Kirchhoff stress here refer to time t is, of course, defined as the same way as the second Piola-Kirchhoff stress as before when it was referred to time 0. But what we have to do now is introduce this t whenever we use the 0 before in the equation linking up the Cauchy stress with the second Piola-Kirchhoff stress.

We already then know the solution at time t. So we know basically these quantities here. And we can, therefore, now decompose directly the stresses into a stress that we know, and an increment. Similarly, we do for the strains. It's now interesting to observe that this stress here is nothing else than the Cauchy stress. If you were to plug in to the formula that I gave you earlier, which once again, expresses the second Piola-Kirchhoff stress in terms of the Cauchy stress, you would simply see that this quantity is nothing else than the Cauchy stress. And therefore, we have that now here. And of course, we carry this part in that equation here.

The Green-Lagrange strain, if written out, one directly identifies that this part here is 0, because we are using only the increments in displacements from time t to time t plus delta t. And we are left with this part here, which is unknown. Let's look at the Green-Lagrange strain here. Notice t plus delta t, t epsilon ij is written as shown here. Where I should point out that we are looking here at the displacements from time t to time t plus delta t.

In other words, if I want to write this out here, I'm doing it as follows. I'm saying that it's a partial of the displacements, t plus delta t, ui minus tui with respect to txj. Notice that here, we are subtracting from the displacement at time t plus delta t, the displacements tui. So it's the incremental displacements that we're dealing with. And we're differentiating with respect to the coordinate at the time t.

Similarly, of course, we define these expressions here, and those expressions, and those expressions. Notice, just to emphasize this point, that this here is nothing else than ui. And if we now substitute from here into there, we directly recognize that this term is, in fact, the same as that one, which I expressed already on the earlier view graph. Notice, linear part here, nonlinear part there. This part here, of course, I like to just write out for you once more. It's nothing else than partial ui with respect to txj.

Notice, here we have a product incremental displacement, and that's why it is, of course, nonlinear. It is interesting to compare this expression for the Green-Lagrange strain, for the incremental Green-Lagrange strain, to the incremental Green-Lagrange strain of the total Lagrangian formulation. And if you do so, you'll find that the major difference is that in the total Lagrangian formulation you have an initial displacement effect. Here, we do not have an initial displacement effect. And in fact, that is one of the advantages of the updated Lagrangian formulation.

We can now define, just as in the total Lagrangian formulation, a linear strain increment, and a nonlinear strain increment. Of course, linear in the incremental displacement of the particles. Nonlinear in the incremental displacement of the particles. And this means that directly we obtain this expression here. And the variation on here, of course, on this total Green-Lagrange strain increment is simply given by these two additions.

The equation of the principle of virtual work now becomes substituting from what I just discussed with you, directly this equation. Notice we have here now, the external virtual work, just as before, and we have here the internal virtual work corresponding to the stresses at time t. And these are actually the Cauchy stresses, the real stresses. This is here again our out of balance virtual work, so to say.

On the left-hand side, we have one part that includes an increment in the stress. Refer to time t now, because the t configuration is our reference configuration. And we are multiplying it by the variation on the total Green-Lagrange strain increment-- that's one integral-- over the volume at time t. And here we have the Cauchy stress times the variation on the nonlinear part of the Green-Lagrange strain increment.

Given a variation in displacement data ui, of course, we can calculate the right-hand side. But we recognize that on the left-hand side, we have unknown displacement increments and there would be nonlinearities occurring on the left-hand side. Remember, that so far no approximations have been made. This is a statement that is valid for any amount of deformation, any amount of displacements, rotations, and strains.

Just as in the total Lagrangian formulation, the equation of the principle of virtual work in the updated Lagrangian formulation is, of course, a very complicated equation and highly nonlinear in the incremental displacement. And we somehow have to linearize. Because we want to obtain a finite element solution, we linearize first the principle of virtual work equation, and then we are ready to obtain an equation as shown here, which is very much like the equation which we are operating on using the total Lagrangian formulation.

Notice, because we're dealing with the updated Lagrangian formulation, we now have a t here, and we have a t there, meaning the reference configuration is the time t configuration, and here as well.

We begin to linearize the terms just as we have done it earlier in the total Lagrangian formulation. And we recognize that this term here is already linear in ui. That is quite simply seen because the stress does not contain ui, it's a known quantity. The variation on this part here gives us this expression. And we recognize that this is linear in ui because here we have the unknown ui appearing. This is going to be a constant for a given variation in displacement. And this is here also linear and ui. This is going to be a constant for a given variation in displacement. And so we have a linear part here in ui. No linearization required here.

Let us now look at the next term. Here we have the increment in the second Piola-Kirchhoff stress times the variation on the increment in the Green-Lagrange strain integrated over the volume at time t. We recognize that tSij is a nonlinear function in general of the increment in the Green-Lagrange strain. If we take the variation on that increment and Green-Lagrange strain, we obtain these two terms, and we recognize that this is also a linear function of ui. Therefore, in this product, we have to neglect now all higher order terms in ui.

First we recognize now that tSij can be written as a Taylor series expansion, as shown here. Notice that tSij is equal to one first term plus higher order terms. These higher order terms we neglect. If you look at this term here, we recognize that we can substitute for this term, as shown here. And this one here is, of course, the constitutive relation, the tangent constitutive relation for the material.

Recognizing that this one here is quadratic in ui, we drop that term and we get this linearized result. Notice that here we have a constitutive tensor referred to the configuration at time t. In the total Lagrangian formulation we had here a 0 instead of a t.

Well if we now look at the product that we wanted to linearize again, we have this product on the left-hand side, which is now approximately equal to this term here. This already we derived as a linearization to that term. Here we are substituting for that term here.

Notice that if we multiply out, we directly obtain this relationship here. This one here does not contain ui of course. And this one here is linear in ui. This means that since this one also is linear in ui, that we have here a quadratic term in ui. Notice this term now, totally linear, this one quadratic, we drop that one, and we are left with the linearized result, the result that we wanted to obtain.

The final linearized equation is then given on this view graph. Notice we have here the constitutive relation times the linear strain increment in the total Green-Lagrange strain increment, the variation on it. And of course, this one again integrated over the volume at time t. Here we have a term where there was no linearization necessary. And here we have a term also there was no linearization necessary. These two terms were obtained already earlier. These here, this term be obtained by linearization.

Notice that when we now use finite element interpolations, we will express this total term here via this term here where we now have a tangent finite element matrix, which is denoted by the t up there at time t referred to the configuration at time t. This t here refers to the configuration at time t. There's an increment in the displacement that we want to solve for, the real displacement increment. And this is here, the virtual displacement vector.

The right-hand side gives us a virtual displacement vector, of course, transposed times this vector, and here we have the externally applied loads coming from the external virtual work minus the nodal point force vector corresponding to the internal element stresses. That is this term here results into this nodal point force vector corresponding to the internal element stresses.

So here we have the continuum mechanics relation in black, linearized from the general principle of virtual work, and here we have the finite element expression of that. Of course, the actual finite element solution is then obtained by actually setting this vector here equal to the identity matrix so that we are left with K times delta U is equal to t plus delta tR minus tF.

An important point in this development is to recognize that this term here is, of course, the virtual work due to the element internal stresses at time t. And we interpret this part here as the out of balance virtual work just in the same way as we have been doing it in the total Lagrangian formulation.

Let's look now at the total solution using the updated Lagrangian formulation. The displacement iteration is much the same way as in the total Lagrangian formulation. We take the old value corresponding to k minus 1 iteration, and add an increment that we are solving for to obtain a new estimate on the displacements. k being the iteration counter. Notice, of course, in this iteration, we have to have initial conditions, and these initial conditions are given right here. In other words, when k is equal to 1, we would have here t plus delta t ui 0, and therefore we need this value, and that value is given right here as t plus delta tui, 0 equal to tui. In other words, the displacement at the configuration t.

If you look now at the total continuum mechanics equations that we are operating on, I should say one continuum mechanics equation really, which, of course, gives us then in the finite element discretization, the set of simultaneous equations that we will be working with. This continuum mechanics equation looks as follows. On the left-hand side we have the tangent constitutive relationship times an increment in real strains that we want to solve for in iteration k. These are the virtual strains.

We integrate that product over the volume at time t. That, of course, holds for any one of these integrals. And here we have now the actual stresses, the real stresses that we know already, which correspond to the configuration at time t. Then a variation on the incremental Green-Lagrange strain, but I should add its only the nonlinear increment in the Green-Lagrange strain corresponding to iteration k. Once again, integrated over the volume at time t. And on the right-hand side, we have the external virtual work and this expression.

Let's look at this expression carefully. Here we have an integral over the volume at time t plus delta t in the iteration k minus 1. Therefore, we are updating that volume as we go along in the iteration. Notice that here we have the stresses corresponding to iteration k minus 1. We are updating these stresses, of course, also in the iteration. Here we have the virtual strains corresponding to iteration at the end of iteration k minus 1. We are updating this as well. And this product here, once again, is integrated over the volume at time t plus delta t corresponding to the end of iteration k minus 1 or the beginning of iteration k.

Well it's important to realize that when this right-hand side is 0, we have fulfilled, or we have basically solved, the equation of the principle of virtual work, because the external virtual work is equal to the internal virtual work. And then, of course, we have obtained the solution. So we iterate with k equals 1, 2, 3, and so on, until the right-hand side is 0, and then we are satisfying that the external virtual work is equal to the internal virtual work.

When we discretize this continuum mechanics equation via finite elements, we obtain this equation here. ttK, once again, the tangent stiffness matrix. Here, the increment in the nodal point displacements corresponding to iteration k. On the right-hand side the R vector of externally applied loads. And here, the nodal point force vector corresponding to the internal element stresses at time t plus delta t in iteration k minus 1. At the end of iteration k minus 1 is more precisely said.

Notice that we are having a t plus delta t down here because we are always updating the actual configuration of reference. Here we are updating the configuration of reference because we are working with the Cauchy stresses at time t plus delta t, and in iteration at the end of iteration k minus 1.

Of course, this vector is calculated from the displacement at time t plus delta t, and iteration k minus 1. These, of course, are known. This is a known quantity, whereas the unknown quantity are the increments in the displacements. These we want to calculate.

We iterate until, with k equals 1, 2, 3, and so on, until the right-hand side is 0. Of course, this is nothing else than an expression of, in finite element analysis, of the principle of virtual work where we had also the right-hand side, this was the external virtual work, and this was the internal virtual work corresponding to the current element stresses.

Notice that the displacements that we are talking about here are obtained by adding all the incremental displacement that we're dealing with here. We are taking these and add them all up as expressed in this summation sign here. j goes from 1 to k to get, and we take that sum, of course, and add it to tU, the displacement corresponding to configuration time t, to get the displacements corresponding to the last iteration.

The total iterative process is summarized on this view graph. We start with a given tU, the displacement at time t are given, and the external loads are also given corresponding to time t plus delta t. We compute the tangent stiffness matrix corresponding to time t, and the nodal point force vector corresponding to the internal element stresses at time t. This nodal point force vector provides initial conditions for this vector, because that is the vector which will enter actually into the iteration.

This displacement vector provides the initial conditions for that vector. We set the iteration counter k equal to 1. We go in here, and notice now we are solving this equation, which we just discussed. When k is equal to 1, of course, we have here F0, and that F0 is nothing else than that vector here. Because that's how we initialized it.

So we can calculate this incremental displacement vector. We add that increment and displacement vector to the displacement which we had already. When k is equal to 1, of course, this vector here is nothing else than that vector, which we are given already. We add on and get a new estimate for the displacements.

We then check for convergence. This means we are checking whether equilibrium is satisfied sufficiently, meaning whether the right-hand side is 0 with this new displacement estimate. If we have not converged, we go into the next calculation of the force vector, the nodal point force vector corresponding to the current internal element stresses. And notice that, of course, now this force vector, these elements stresses that go in here, are computed based on the displacement at time t plus delta t at the end of iteration k.

In other words, these are the displacements that we have just been calculating right there. We increase the iteration counter, and keep on looping as before. So this is the loop that we go through in the iteration until we converge, until we converge.

If you compare this view graph with the view graph that we discussed in the total Lagrangian formulation, you will find a great similarity. You will find that basically all of these quantities have already been discussed in the total Lagrangian formulation, except that in the total Lagrangian formulation we always carry it down here and down there as 0 instead of t, t plus delta t here. We carry it as 0.

Well because the reference configuration was the 0 configuration. Now the reference configuration for the stiffness matrix is the t configuration. The reference configuration for the F vector is the t plus delta t configuration after iteration k minus 1.

So there's a great similarity in the iteration process, in the finite element matrices that we are setting up. And of course, we have to ask ourselves what comparison is there between the T.L. and U.L. formulation? Why would one want to use the U.L. formulation if we have the T.L. formulation, for example.

Well in the T.L. formulation, all the derivatives are defined with respect to the original configuration. In the U.L. formulation, they are defined with respect to the current configuration. And that will provide differences, that does provide differences. For example, in the U.L. formulation, we will have to calculate the derivatives newly in each iteration, because the configuration is continuously updated. We don't need to do it, of course, for the k matrix if we keep the k matrix constant on the left-hand side.

But for the F vector on the right-hand side, we will have to always calculate new derivatives because the F vector involves the Cauchy stresses times the infinitesimal virtual strains referred to the current configuration, t plus delta t, iteration k minus 1, which is being updated. And so we would always calculate new derivatives right there.

There, of course, is an expense involved. On the other hand, the U.L. formulation does not have this initial displacement effect that I mentioned earlier, so there's an advantage. In the U.L. formulation, we also directly deal with Cauchy stresses.

These are, of course, the physical stresses that we are actually interested in, and that we want to get printed out from the computer program. Hence, there's no transformation necessary from some stress measure, and I'm thinking of the second Piola-Kirchhoff stress that we use in the total Lagrangian formulation, to the Cauchy stresses that we actually want to obtain in the computer program, and that we as engineers want to get, of course, printed out.

Well if we now look at one other important point, one important point, regarding the T.L. and U.L. formulation, we recognize that if we make the assumption, the same assumptions, in both of these two formulations in the linearization, as we, of course, have done, really, the way I've presented it. Then the U.L. and T.L. formulation, provided the appropriate transformations are made, certain transformation rules are followed for the stresses, the strains, and so on. Those rules we will be talking about further later on in later lectures. Then, indeed, exactly the same stiffness matrix and force vector, F vector, vectors I should say, are calculated.

In other words, provided we follow certain transformation rules, indeed, the same stiffness matrices and force vectors are calculated in both of these formulations. Of course, the actual numerical arithmetic required to obtain those matrices and vectors are, of course, different. But the final result is the same.

We can demonstrate that in the general case, as I will do later on in a lecture, and we can also demonstrate that on simple examples. In fact, we will look in one lecture at the truss element where we can directly show that the T.L. and U.L. formulation really give exactly the same result for the k matrix, as well as the F vector. So there we have an example, but as I mentioned, we can also show it in the general case, and that we do as well later on.

Thank you very much for your attention.

Free Downloads

Video

• iTunes U (MP4 - 110MB)
• Internet Archive (MP4 - 110MB)

Subtitle

• English - US (SRT)