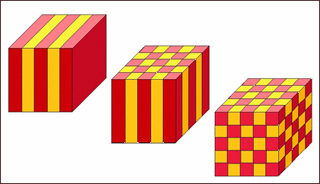
Photonic crystals are arrangements of two or more dielectric media, periodic along one, two, or three dimensions. This is depicted schematically here by "red" and "yellow" materials arranged in simple 1d/2d/3d lattices from left to right. (Image by Prof. Johnson.)
Instructor(s)
Prof. Steven G. Johnson
MIT Course Number
18.369
As Taught In
Spring 2008
Level
Graduate
Course Description
Course Features
Course Description
Find out what solid-state physics has brought to Electromagnetism in the last 20 years. This course surveys the physics and mathematics of nanophotonics—electromagnetic waves in media structured on the scale of the wavelength.
Topics include computational methods combined with high-level algebraic techniques borrowed from solid-state quantum mechanics: linear algebra and eigensystems, group theory, Bloch's theorem and conservation laws, perturbation methods, and coupled-mode theories, to understand surprising optical phenomena from band gaps to slow light to nonlinear filters.
Note: An earlier version of this course was published on OCW as 18.325 Topics in Applied Mathematics: Mathematical Methods in Nanophotonics, Fall 2005.
Other Versions
Other OCW Versions
Archived versions: ![]()


